Page 105 - Maths Skills - 8

Linear Equations in One Variable 103

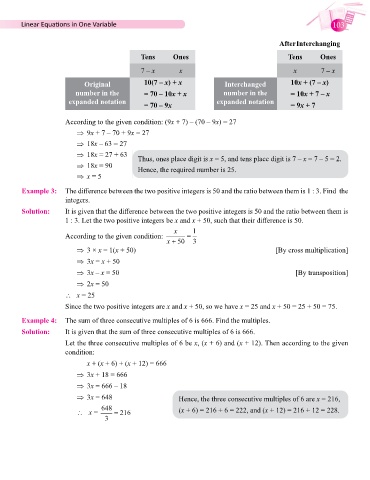
After Interchanging

Tens Ones Tens Ones

7 – x x x 7 – x
Original 10(7 – x) + x Interchanged 10x + (7 – x)
number in the = 70 – 10x + x number in the = 10x + 7 – x
expanded notation expanded notation
= 70 – 9x = 9x + 7

According to the given condition: (9x + 7) – (70 – 9x) = 27
⇒ 9x + 7 – 70 + 9x = 27
⇒ 18x – 63 = 27

⇒ 18x = 27 + 63 Thus, ones place digit is x = 5, and tens place digit is 7 – x = 7 – 5 = 2.
⇒ 18x = 90 Hence, the required number is 25.
⇒ x = 5

Example 3: The difference between the two positive integers is 50 and the ratio between them is 1 : 3. Find the
integers.
Solution: It is given that the difference between the two positive integers is 50 and the ratio between them is
1 : 3. Let the two positive integers be x and x + 50, such that their difference is 50.

x 1
According to the given condition:
x 50 3
⇒ 3 × x = 1(x + 50) [By cross multiplication]

⇒ 3x = x + 50
⇒ 3x – x = 50 [By transposition]

⇒ 2x = 50
∴ x = 25
Since the two positive integers are x and x + 50, so we have x = 25 and x + 50 = 25 + 50 = 75.

Example 4: The sum of three consecutive multiples of 6 is 666. Find the multiples.
Solution: It is given that the sum of three consecutive multiples of 6 is 666.

Let the three consecutive multiples of 6 be x, (x + 6) and (x + 12). Then according to the given
condition:
x + (x + 6) + (x + 12) = 666
⇒ 3x + 18 = 666
⇒ 3x = 666 – 18

⇒ 3x = 648 Hence, the three consecutive multiples of 6 are x = 216,

∴ x = 648 = 216 (x + 6) = 216 + 6 = 222, and (x + 12) = 216 + 12 = 228.
3