Page 199 - Maths Skills - 7

Data Handling 197

Now, from the above data.

Lowest value = 0 and highest value = 49
Thus, Range = 49 – 0 = 49

MEDIAN
The value of the middle observation, after arranging the given data in an ascending or a descending order of
magnitude is called the median of the data.
In the above observations, arranging the data in ascending order, we get 0, 15, 25, 25, 35, 38, 45, 49, 49, 49.
Here, the total number of observations are ten (an even number), hence, the median will be the average of 5th and
6th value, i.e.,
35 + 38 73
Median = = = 36.5
2 2
Thus, we conclude that median of an ungrouped data can be found in the following way:

Step 1. Arrange the data in increasing or decreasing order. Let the total number of observations be n.

 n +  1
Step 2. (i) If n is odd, median =   th value.
 2 

n

1    n  
(ii) If n is even, median =    th value +  + 1 th value 

2
2    2  

MODE OF AN UNGROUPED DATA
In the given data, “The value which occurs most frequently is called the mode”.
For example; in the previous discussion the marks scored are:
49, 35, 15, 49, 0, 25, 45, 49, 25, 38 Fact-o-meter
Here, 49 occurs three times. Hence, the mode is 49. If we summarize the When the data is large,
mean, median and mode for the discussed example, we observe that the making a frequency table
mean is 33, the median is 36.5 and the mode is 49. This shows that each is preferred.
measure of central tendency has a different value.
Since the data is large, we convert it into frequency table.
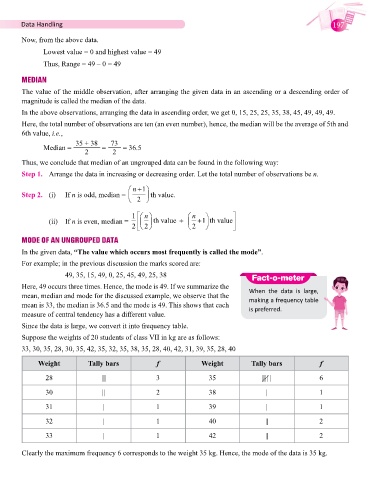
Suppose the weights of 20 students of class VII in kg are as follows:

33, 30, 35, 28, 30, 35, 42, 35, 32, 35, 38, 35, 28, 40, 42, 31, 39, 35, 28, 40

Weight Tally bars f Weight Tally bars f

28 ||| 3 35 |||| | 6

30 || 2 38 | 1

31 | 1 39 | 1

32 | 1 40 || 2

33 | 1 42 || 2

Clearly the maximum frequency 6 corresponds to the weight 35 kg. Hence, the mode of the data is 35 kg.