Page 130 - Maths Skill - 6

128 Maths

INTRODUCTION
Have you ever observed the wings of a butterfly? Imagine, if the design on the wings are different.
How absurd will it be? This shows that the nature also follows symmetry. A design is said to
have symmetry, if you can move the entire design by either rotation, reflection or translation
and the design appears unchanged. Symmetry is a geometrical property that can be identified in
everyday life in natural as well as man-made environment. It can arise in several ways.
In this chapter we will discuss the reflection symmetry which is the most easily recognizable kind of symmetry
and is associated with 2D space and the idea of mirror image.
Let’s do an activity.

Activity
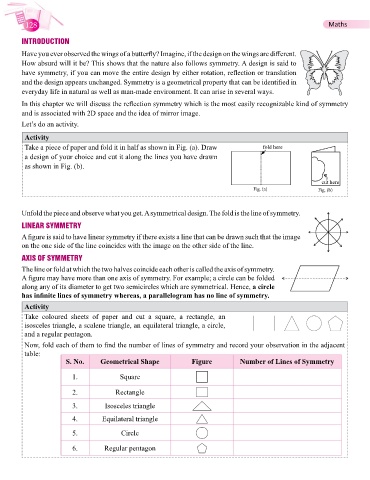
Take a piece of paper and fold it in half as shown in Fig. (a). Draw fold here
a design of your choice and cut it along the lines you have drawn
as shown in Fig. (b).

cut here
Fig. (a) Fig. (b)

Unfold the piece and observe what you get. A symmetrical design. The fold is the line of symmetry.

LINEAR SYMMETRY
A figure is said to have linear symmetry if there exists a line that can be drawn such that the image
on the one side of the line coincides with the image on the other side of the line.
AXIS OF SYMMETRY
The line or fold at which the two halves coincide each other is called the axis of symmetry.
A figure may have more than one axis of symmetry. For example; a circle can be folded
along any of its diameter to get two semicircles which are symmetrical. Hence, a circle
has infinite lines of symmetry whereas, a parallelogram has no line of symmetry.
Activity
Take coloured sheets of paper and cut a square, a rectangle, an
isosceles triangle, a scalene triangle, an equilateral triangle, a circle,
and a regular pentagon.
Now, fold each of them to find the number of lines of symmetry and record your observation in the adjacent
table:
S. No. Geometrical Shape Figure Number of Lines of Symmetry

1. Square

2. Rectangle

3. Isosceles triangle
4. Equilateral triangle

5. Circle

6. Regular pentagon