Page 135 - Maths Skill - 6
P. 135
Symmetry 133
ROTATIONAL SYMMETRY
The rotational symmetry of a shape describes how, when an object is rotated on its own axis, the shape of the
object remains the same.
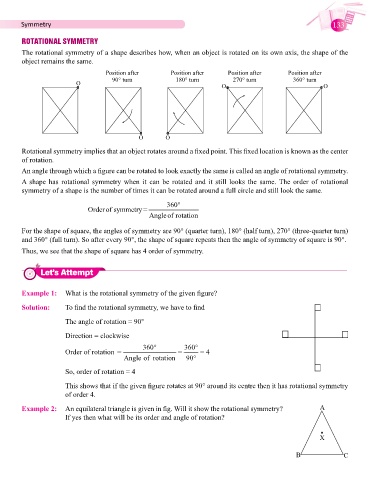
Position afterPosition afterPosition afterPosition afterPosition after
Position afterPosition afterPosition afterPosition afterPosition after
Position afterPosition afterPosition afterPosition afterPosition after Position afterPosition afterPosition afterPosition afterPosition after
180° turn180° turn 180° turn180° turn
270° turn270° turn270° turn
90° turn 90° turn 90° turn90° turn90° turn 180° turn 270° turn 360° turn
360° turn360° turn360° turn360° turn270° turn
O O O O O
O O O O O O O O O O
O OO OO OO O O O
Rotational symmetry implies that an object rotates around a fixed point. This fixed location is known as the center
of rotation.
An angle through which a figure can be rotated to look exactly the same is called an angle of rotational symmetry.
A shape has rotational symmetry when it can be rotated and it still looks the same. The order of rotational
symmetry of a shape is the number of times it can be rotated around a full circle and still look the same.
360°
Orderofsymmetry=
Angleofrotation
For the shape of square, the angles of symmetry are 90° (quarter turn), 180° (half turn), 270° (three-quarter turn)
and 360° (full turn). So after every 90°, the shape of square repeats then the angle of symmetry of square is 90°.
Thus, we see that the shape of square has 4 order of symmetry.
Let’s Attempt
Example 1: What is the rotational symmetry of the given figure?
Solution: To find the rotational symmetry, we have to find
The angle of rotation = 90°
Direction = clockwise
Order of rotation = 360° = 360° =4
Angleofrotation 90°
So, order of rotation = 4
This shows that if the given figure rotates at 90° around its centre then it has rotational symmetry
of order 4.
Example 2: An equilateral triangle is given in fig. Will it show the rotational symmetry? A
If yes then what will be its order and angle of rotation?
X
B C