Page 139 - Maths Skill - 6

Symmetry 137

HOTS

1. How many lines of symmetry are there in digit 3, digit 0 and numeral 30? What do you observe?
2. How many lines of symmetry does a trapezium have? Which trapezium has one line of symmetry?

3. Can a line of symmetry be called reflection symmetry? Justify or contradict with an example.
4. Which line acts as the axis of symmetry for an angle with equal arms? What if the arms are unequal?

Word Stock
Symmetry Mirror-image Reflation Rotational Linear

Activity

Objective: To understand the rotational symmetry and apply it to create design.
Pre-Requisite Knowledge: Concepts of symmetry and rotation.

Materials Required: Glazed paper, tracing paper, a pencil, an eraser and pins
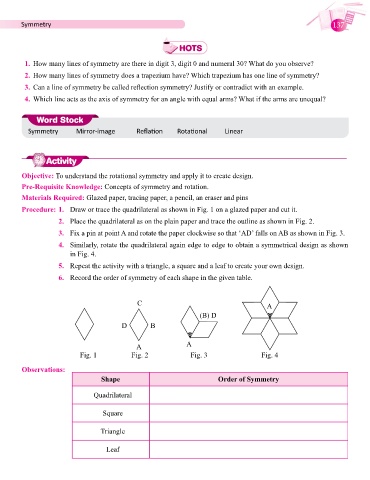
Procedure: 1. Draw or trace the quadrilateral as shown in Fig. 1 on a glazed paper and cut it.
2. Place the quadrilateral as on the plain paper and trace the outline as shown in Fig. 2.

3. Fix a pin at point A and rotate the paper clockwise so that ‘AD’ falls on AB as shown in Fig. 3.
4. Similarly, rotate the quadrilateral again edge to edge to obtain a symmetrical design as shown
in Fig. 4.

5. Repeat the activity with a triangle, a square and a leaf to create your own design.
6. Record the order of symmetry of each shape in the given table.

C A
(B) D
D B

A A
Fig. 1 Fig. 2 Fig. 3 Fig. 4

Observations:
Shape Order of Symmetry

Quadrilateral

Square

Triangle

Leaf