Page 124 - Maths Skill - 6

122 Maths

EXPLORATION IN RECTANGLES
To understand this topic “Exploration in Rectangles” on behalf of the sides or length between any two given
points, we have to construct a rectangle with sides equal to 8 cm and 4 cm.
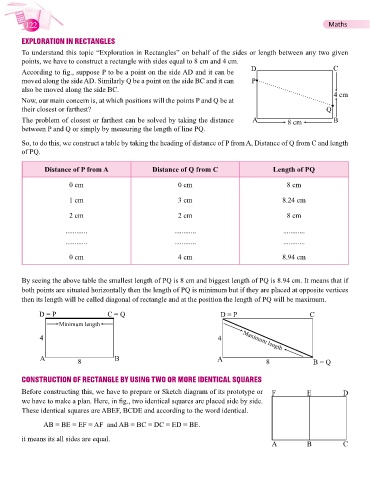
According to fig., suppose P to be a point on the side AD and it can be D C
moved along the side AD. Similarly Q be a point on the side BC and it can P
also be moved along the side BC.

Now, our main concern is, at which positions will the points P and Q be at 4 cm
their closest or farthest? Q
The problem of closest or farthest can be solved by taking the distance A 8 cm B
between P and Q or simply by measuring the length of line PQ.
So, to do this, we construct a table by taking the heading of distance of P from A, Distance of Q from C and length
of PQ.

Distance of P from A Distance of Q from C Length of PQ

0 cm 0 cm 8 cm

1 cm 3 cm 8.24 cm

2 cm 2 cm 8 cm

............ ............ ............

............ ............ ............

0 cm 4 cm 8.94 cm

By seeing the above table the smallest length of PQ is 8 cm and biggest length of PQ is 8.94 cm. It means that if
both points are situated horizontally then the length of PQ is minimum but if they are placed at opposite vertices
then its length will be called diagonal of rectangle and at the position the length of PQ will be maximum.

D = P C = Q D = P D = P C C
Minimum length

4 4 Minimum length 4 Maximum length

A B A A
8 8 B = Q 8 B = Q

CONSTRUCTION OF RECTANGLE BY USING TWO OR MORE IDENTICAL SQUARES

Before constructing this, we have to prepare or Sketch diagram of its prototype or F E D
we have to make a plan. Here, in fig., two identical squares are placed side by side.
These identical squares are ABEF, BCDE and according to the word identical.
AB = BE = EF = AF and AB = BC = DC = ED = BE.

it means its all sides are equal.
A B C