Page 64 - Math Skill - 5

62 Maths

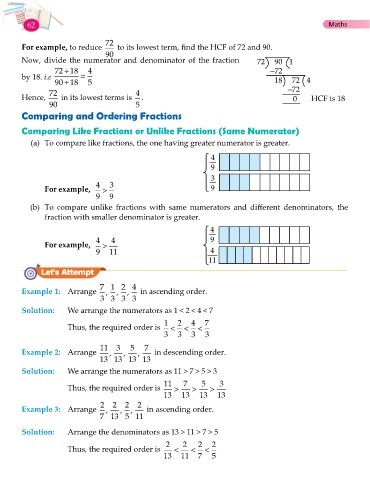
For example, to reduce 72 to its lowest term, find the HCF of 72 and 90.
90
Now, divide the numerator and denominator of the fraction 72 90 1

72 18÷ 4 –72
by 18. i.e =
72 4
90 18÷ 5 18 –72
4
Hence, 72 in its lowest terms is . 0 HCF is 18
90 5

Comparing and Ordering Fractions

Comparing Like Fractions or Unlike Fractions (Same Numerator)
(a) To compare like fractions, the one having greater numerator is greater.

4
9

3
4 3
For example, > 9
9 9

(b) To compare unlike fractions with same numerators and different denominators, the
fraction with smaller denominator is greater.
4

For example, 4 > 4 9
9 11 4
11

Let’s Attempt

7 1 2 4
Example 1: Arrange , , , in ascending order.
3 3 3 3

Solution: We arrange the numerators as 1 < 2 < 4 < 7

Thus, the required order is < 1 2 < 4 < 7
3 3 3 3
11 3 5 7
Example 2: Arrange , , , in descending order.
13 13 13 13
Solution: We arrange the numerators as 11 > 7 > 5 > 3

11 7 5 3
Thus, the required order is > > >
13 13 13 13
2 2 2 2
Example 3: Arrange , , , in ascending order.
7 13 5 11

Solution: Arrange the denominators as 13 > 11 > 7 > 5
2 2 2 2
Thus, the required order is < < <
13 11 7 5