Page 87 - Mathematics Class - XII

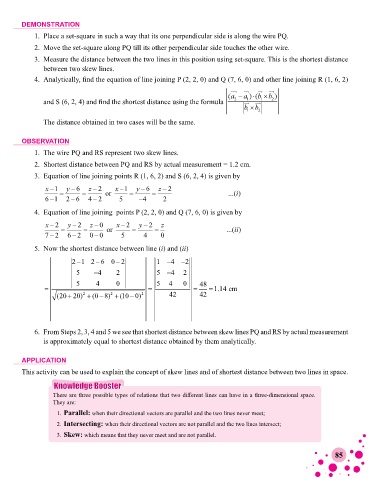
DEMONSTRATION
1. Place a set-square in such a way that its one perpendicular side is along the wire PQ.

2. Move the set-square along PQ till its other perpendicular side touches the other wire.
3. Measure the distance between the two lines in this position using set-square. This is the shortest distance
between two skew lines.
4. Analytically, find the equation of line joining P (2, 2, 0) and Q (7, 6, 0) and other line joining R (1, 6, 2)

(a a )
) (bb
and S (6, 2, 4) and find the shortest distance using the formula 2 1 2
1

bb
1 2
The distance obtained in two cases will be the same.
OBSERVATION
1. The wire PQ and RS represent two skew lines.

2. Shortest distance between PQ and RS by actual measurement = 1.2 cm.
3. Equation of line joining points R (1, 6, 2) and S (6, 2, 4) is given by

x 1 y 6 z 2 or x 1 y 6 z 2 ...( i)

61 26 42 5 4 2
4. Equation of line joining points P (2, 2, 0) and Q (7, 6, 0) is given by
x 2 y 2 z 0 or x 2 y 2 z ...( ii)

72 62 00 5 4 0
5. Now the shortest distance between line (i) and (ii)

2 126 02 1 4 2
5 4 2 5 4 2
5 4 0 5 4 0 48
114. cm

(
(
( 20 20) 2 08) 2 10 0) 2 42 4 42

6. From Steps 2, 3, 4 and 5 we see that shortest distance between skew lines PQ and RS by actual measurement
is approximately equal to shortest distance obtained by them analytically.

APPLICATION

This activity can be used to explain the concept of skew lines and of shortest distance between two lines in space.
Knowledge Booster

There are three possible types of relations that two different lines can have in a three-dimensional space.
They are:
1. Parallel: when their directional vectors are parallel and the two lines never meet;

2. Intersecting: when their directional vectors are not parallel and the two lines intersect;
3. Skew: which means that they never meet and are not parallel.

85