Page 91 - Mathematics Class - XII

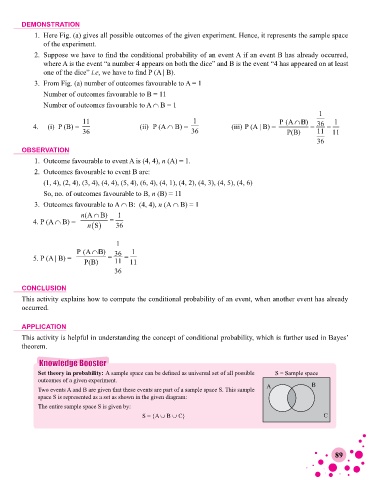
DEMONSTRATION
1. Here Fig. (a) gives all possible outcomes of the given experiment. Hence, it represents the sample space
of the experiment.
2. Suppose we have to find the conditional probability of an event A if an event B has already occurred,
where A is the event “a number 4 appears on both the dice” and B is the event “4 has appeared on at least
one of the dice” i.e, we have to find P (A | B).
3. From Fig. (a) number of outcomes favourable to A = 1
Number of outcomes favourable to B = 11

Number of outcomes favourable to A ∩ B = 1
1
4. (i) P (B) = 11 (ii) P (A ∩ B) = 1 (iii) P (A | B) = P A( B ) 36 1
36 36 P(B) 11 11
36
OBSERVATION
1. Outcome favourable to event A is (4, 4), n (A) = 1.
2. Outcomes favourable to event B are:

(1, 4), (2, 4), (3, 4), (4, 4), (5, 4), (6, 4), (4, 1), (4, 2), (4, 3), (4, 5), (4, 6)
So, no. of outcomes favourable to B, n (B) = 11
3. Outcomes favourable to A ∩ B: (4, 4), n (A ∩ B) = 1
n(A ) B 1
4. P (A ∩ B) = n S 36

1
P A( B ) 1
5. P (A | B) = 36
PB() 11 11
36

CONCLUSION

This activity explains how to compute the conditional probability of an event, when another event has already
occurred.

APPLICATION
This activity is helpful in understanding the concept of conditional probability, which is further used in Bayes’
theorem.

Knowledge Booster
Set theory in probability: A sample space can be defined as universal set of all possible S = Sample space
outcomes of a given experiment. B
Two events A and B are given that these events are part of a sample space S. This sample A
space S is represented as a set as shown in the given diagram:
The entire sample space S is given by:
S = {A ∪ B ∪ C} C

89