Page 37 - Mathematics Class - XII

y
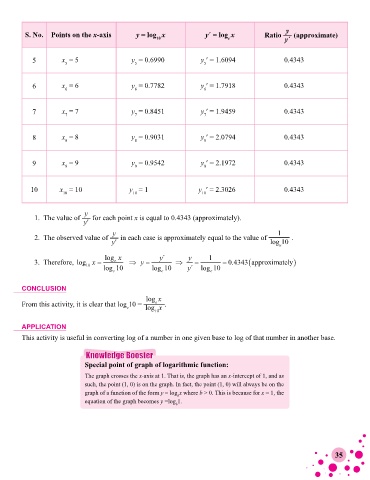
S. No. Points on the x-axis y = log x y´ = log x Ratio (approximate)
10 e y´

5 x = 5 y = 0.6990 y ′ = 1.6094 0.4343
5
5
5

6 x = 6 y = 0.7782 y ′ = 1.7918 0.4343
6
6
6
7 x = 7 y = 0.8451 y ′ = 1.9459 0.4343
7
7
7
8 x = 8 y = 0.9031 y ′ = 2.0794 0.4343
8
8
8

9 x = 9 y = 0.9542 y ′ = 2.1972 0.4343
9
9
9
10 x = 10 y = 1 y ′ = 2.3026 0.4343
10
10
10
y
1. The value of for each point x is equal to 0.4343 (approximately).
y′
y 1
2. The observed value of in each case is approximately equal to the value of .
y′ log 10
e
log x ´ y y 1

3. Therefore, log x e y . 0 4343 approximateely
10
log 10 log 10 ´ y log 10
e
e
e
CONCLUSION
log x
From this activity, it is clear that log 10 = e .
e log x
10
APPLICATION

This activity is useful in converting log of a number in one given base to log of that number in another base.

Knowledge Booster
Special point of graph of logarithmic function:

The graph crosses the x-axis at 1. That is, the graph has an x-intercept of 1, and as
such, the point (1, 0) is on the graph. In fact, the point (1, 0) will always be on the
graph of a function of the form y = log x where b > 0. This is because for x = 1, the
b
equation of the graph becomes y =log 1.
b

35