Page 33 - Mathematics Class - XII

Viva-Voce

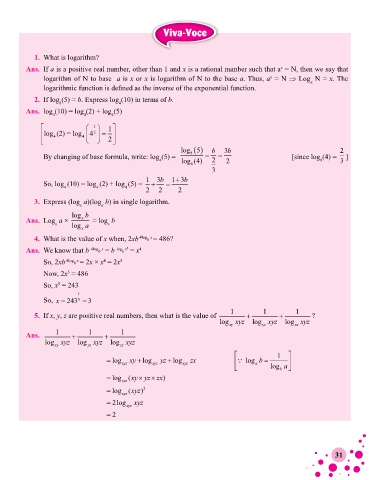
1. What is logarithm?
Ans. If a is a positive real number, other than 1 and x is a rational number such that a = N, then we say that
x
logarithm of N to base a is x or x is logarithm of N to the base a. Thus, a = N ⇒ Log N = x. The
x
a
logarithmic function is defined as the inverse of the exponential function.
2. If log (5) = b. Express log (10) in terms of b.
8 4
Ans. log (10) = log (2) + log (5)
4 4 4
1 1

log(2) = log 4 4 2
4
2

log 5 b 3b 2
By changing of base formula, write: log (5) = 8 [since log (4) = ]
4 log( )4 2 2 8 3
8
3
1
So, log (10) = log (2) + log (5) = 3b 13 b
4 4 4
2 2 2
3. Express (log a)(log b) in single logarithm.
x a
log b
Ans. Log a × x = log b
x log a x
x
b = 486?
4. What is the value of x when, 2xb 4log x
b = b
b = x
Ans. We know that b 4log x log x 4 4
b = 2x × x = 2x
So, 2xb 4log x 4 5
Now, 2x = 486
5
So, x = 243
5
1
So, x = 243 5 = 3

5. If x, y, z are positive real numbers, then what is the value of 1 + 1 + 1 ?
log xyz log xyz log xyz
xy
zx
yz
Ans. 1 1 1
log xyz log xyz log xyz
xy
yz
zx
1
log xyz xy log xyz yz log xyz zx  loog b
a
log a
b

log(xy yz zx )

xyz
log(xyz ) 2
xyz
2 log xyz xyzz
2

31