Page 28 - Mathematics Class - XII

–p p
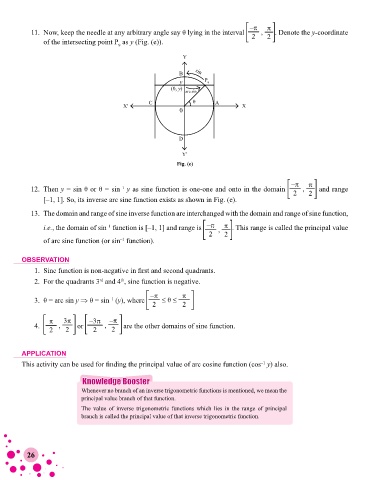
11. Now, keep the needle at any arbitrary angle say θ lying in the interval 2 , 2 . Denote the y-coordinate
of the intersecting point P as y (Fig. (e)).
9
Y

B sin
y P 9
(0, y)
arc sin
C θ A
X′ X
0

D

Y′
Fig. (e)

–p p
12. Then y = sin θ or θ = sin y as sine function is one-one and onto in the domain 2 , 2 and range
–1
[–1, 1]. So, its inverse arc sine function exists as shown in Fig. (e).

13. The domain and range of sine inverse function are interchanged with the domain and range of sine function,

i.e., the domain of sin function is [–1, 1] and range is –p , p . This range is called the principal value
–1
2 2
of arc sine function (or sin function).
–1

OBSERVATION
1. Sine function is non-negative in first and second quadrants.
2. For the quadrants 3 and 4 , sine function is negative.
rd
th
–p p
3. θ = arc sin y ⇒ θ = sin (y), where ≤ θ ≤
–1
2 2
p 3p –3p –p
4. 2 , 2 or 2 , 2 are the other domains of sine function.

APPLICATION
This activity can be used for finding the principal value of arc cosine function (cos y) also.
–1
Knowledge Booster

Whenever no branch of an inverse trigonometric functions is mentioned, we mean the
principal value branch of that function.
The value of inverse trigonometric functions which lies in the range of principal
branch is called the principal value of that inverse trigonometric function.

26