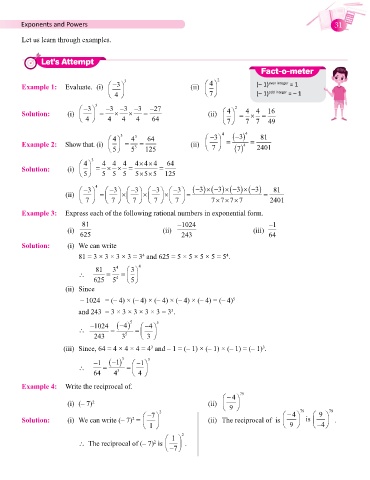
Page 33 - Maths Skills - 8

Exponents and Powers 31

Let us learn through examples.

Let’s Attempt
Fact-o-meter

Example 1: Evaluate. (i) 3 3 (ii) 4 2 (– 1) even integer = 1
(– 1) odd integer = – 1
4 7

Solution: (i) 3 3 3 3 3 27 (ii) 4 2 16
4 4

4 4 4 4 64 7 7 49
7

4 3 4 3 64  −  3 4 = − ( ) 3 4 = 81
Example 2: Show that. (i) (ii)    4
5 5 3 125  7 () 7 2401

4 3 4 4 4 444 64
Solution: (i)

5 5 5 5 555 125

3
3
3
(ii) 3 4 3 3 3 3 3 81

7 7 7 7 7 7777 2401
Example 3: Express each of the following rational numbers in exponential form.
81 −1024 −1
(i) (ii) (iii)
625 243 64
Solution: (i) We can write
81 = 3 × 3 × 3 × 3 = 3 and 625 = 5 × 5 × 5 × 5 = 5 .
4
4
3
\ 81 3 4 4
625 5 4 5
(ii) Since
– 1024 = (– 4) × (– 4) × (– 4) × (– 4) × (– 4) = (– 4) 5
and 243 = 3 × 3 × 3 × 3 × 3 = 3 .
5
5 5

\ 1024 4 4

243 3 5 3
(iii) Since, 64 = 4 × 4 × 4 = 4 and – 1 = (– 1) × (– 1) × (– 1) = (– 1) .
3
3
3 3
1
\ 1 1
64 4 3 4
Example 4: Write the reciprocal of.

4 79
(i) (– 7) 2 (ii)
9 79 79
2

4
Solution: (i) We can write (– 7) = 7 (ii) The reciprocal of is is 9
.

2

1 9 4
1 2
\ The reciprocal of (– 7) is .
2
7