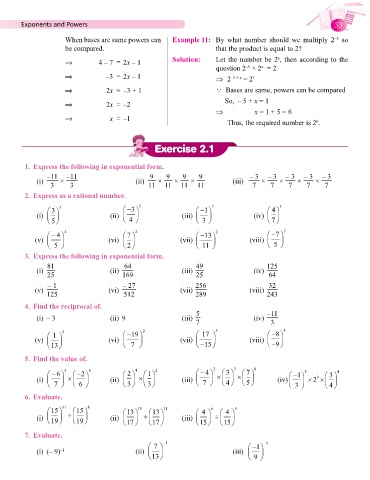
Page 35 - Maths Skills - 8

Exponents and Powers 33

When bases are same powers can Example 11: By what number should we multiply 2 so
–5
be compared. that the product is equal to 2?
x
⇒ 4 – 7 = 2x – 1 Solution: Let the number be 2 , then according to the
question 2 × 2 = 2
–5
x
⇒ –3 = 2x – 1 ⇒ 2 –5 + x = 2 1
⇒ 2x = –3 + 1  Bases are same, powers can be compared
⇒ 2x = –2 So, – 5 + x = 1
⇒ x = 1 + 5 = 6
⇒ x = –1
Thus, the required number is 2 .
6

Exercise 2.1

1. Express the following in exponential form.
–11 –11 9 9 9 9 – 3 – 3 – 3 – 3 – 3
(i) × (ii) × × × (iii) × × × ×
3 3 11 11 11 11 7 7 7 7 7
2. Express as a rational number.

3 3 3 5 1 7 4 3
(i) (ii) (iii) (iv)
5 4 3 7

7
(v) 4 4 (vi) 7 2 (vii) 13 2 (viii) 5

5 2 11 5
3. Express the following in exponential form.
81 64 49 125
(i) 25 (ii) 169 (iii) 25 (iv) 64
– 1 – 27 256 32
(v) 125 (vi) 512 (vii) 289 (viii) 243
4. Find the reciprocal of.
(i) – 3 (ii) 9 (iii) 5 (iv) –11
7 3

8
(v) 1 4 (vi) 19 2 (vii) 17 3 (viii) 5

13 7 15 9
5. Find the value of.

7

3
6 5 2 6 2 4 1 2 4 2 3 8 1 5 4
3
(i) (ii) (iii) (iv) 2 3
4
5
7 6 3 3 7 3
4
6. Evaluate.
15 11 15 8 13 15 13 11 4 6 4 9

(i) 19 19 (ii) 17 17 (iii) 15 15

7. Evaluate.
7 1 1 1
(i) (– 9) –1 (ii) (iii)
13 9