Page 218 - Maths Skills - 8

216 Maths

Exercise 13.2

1. Draw the graph between simple interest versus time when the principal is 4000 and the rate of interest
is 3% per annum.

2. Draw the graph between area of circle and its radius.
3. Using the following data of the temperature at 12 o’clock during a certain week. Plot the graph between
temperature versus day.

Day Mon Tue Wed Thu Fri Sat Sun
Temperature (in °C) 35°C 34°C 37°C 39°C 42°C 36°C 40°C

LINEAR GRAPHS
A linear graph is obtained when the coordinates are joined to form a straight line. The general form of a linear graph
is represented as y = mx + c, also called a linear function. Here y and x are the two variables, m is the coefficient
of ‘x’ which may take any value depending on the conditions and ‘c’ is the constant also called intercept.

Distance-Time Graph
The graphs used to describe motion of a vehicle such as a cycle, car, train, aeroplane, etc. are called travel graphs
or distance-time graphs. The distance travelled is represented on y-axis and the time taken on the x-axis, i.e.,
time is an independent variable and distance travelled is a dependent variable.

These graphs can be used to find the speed of the vehicle. Let’s take an example to understand the topic further.
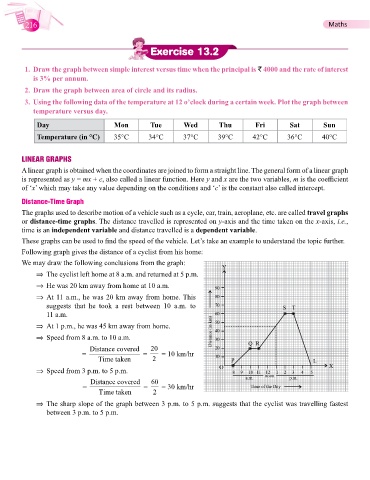
Following graph gives the distance of a cyclist from his home:
We may draw the following conclusions from the graph:

⇒ The cyclist left home at 8 a.m. and returned at 5 p.m.
⇒ He was 20 km away from home at 10 a.m.
⇒ At 11 a.m., he was 20 km away from home. This
suggests that he took a rest between 10 a.m. to
11 a.m.
⇒ At 1 p.m., he was 45 km away from home.
⇒ Speed from 8 a.m. to 10 a.m.

= Distance covered = 20 = 10 km/hr
Time taken 2
⇒ Speed from 3 p.m. to 5 p.m.

= Distance covered = 60 = 30 km/hr
Time taken 2
⇒ The sharp slope of the graph between 3 p.m. to 5 p.m. suggests that the cyclist was travelling fastest
between 3 p.m. to 5 p.m.