Page 215 - Maths Skills - 8

Introduction to Graphs 213

2. Plot the following set of points on the separate graph:

(i) (3, 0), (–3, 2), (4, –7) (ii) (0, –6), (–2, 5), (3, 1), (–3, –5)
3. In which quadrant would the following points be found:
(i) (1, 1) (ii) (1, 2) (iii) (2, 1)

(iv) (–1, 2) (v) (439, –890) (vi) (–1, –1)
4. Plot each set of points on a different plane and join them in order to form a quadrilateral. Identify the
quadrilateral.
(i) A(1, 1), B(1, 5), C(3, 5), D(3, 1) (ii) J(1, 3), K(5, 1), L(8, 1), M(4, 3)

(iii) W(1, 1), X(0, 3), Y(4, 1), Z(3, 1)
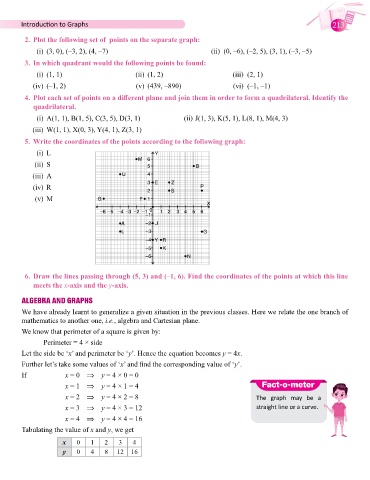
5. Write the coordinates of the points according to the following graph:
(i) L

(ii) S
(iii) A

(iv) R
(v) M

6. Draw the lines passing through (5, 3) and (–1, 6). Find the coordinates of the points at which this line
meets the x-axis and the y-axis.

ALGEBRA AND GRAPHS
We have already learnt to generalize a given situation in the previous classes. Here we relate the one branch of
mathematics to another one, i.e., algebra and Cartesian plane.
We know that perimeter of a square is given by:

Perimeter = 4 × side
Let the side be ‘x’ and perimeter be ‘y’. Hence the equation becomes y = 4x.
Further let’s take some values of ‘x’ and find the corresponding value of ‘y’.
If x = 0 ⇒ y = 4 × 0 = 0
x = 1 ⇒ y = 4 × 1 = 4 Fact-o-meter

x = 2 ⇒ y = 4 × 2 = 8 The graph may be a
x = 3 ⇒ y = 4 × 3 = 12 straight line or a curve.
x = 4 ⇒ y = 4 × 4 = 16

Tabulating the value of x and y, we get
x 0 1 2 3 4
y 0 4 8 12 16