Page 67 - Maths Skills - 7

Rational Numbers 65

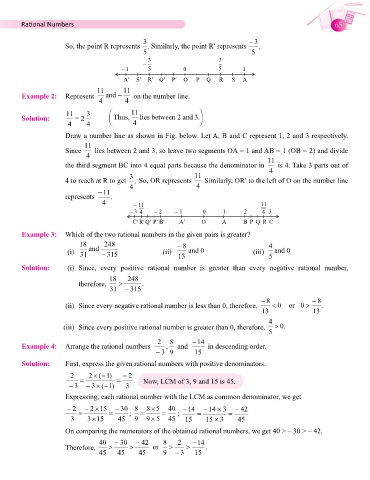
3 − 3
So, the point R represents . Similarly, the point R′ represents .
5 5
3 3
−
– 1 5 0 5 1
A′ S′ R′ Q′ P′ O P Q R S A
11 11
Example 2: Represent and − on the number line.
4 4
11 3  11 
Solution: =2  Thus, liesbetween 2and 3. 


4 4 4
Draw a number line as shown in Fig. below. Let A, B and C represent 1, 2 and 3 respectively.
11
Since lies between 2 and 3, so leave two segments OA = 1 and AB = 1 (OB = 2) and divide
4 11
the third segment BC into 4 equal parts because the denominator in 4 is 4. Take 3 parts out of
3 11
4 to reach at R to get . So, OR represents 4 Similarly, OR′ to the left of O on the number line

−11 4
represents .
4 – 11 11
– 3 4 – 2 – 1 0 1 2 4 3
C′ R′ Q′ P′ B′ A′ O A B P Q R C
Example 3: Which of the two rational numbers in the given pairs is greater?
18 248 − 8 4
(i) 31 and − 315 (ii) and 0 (iii) and 0
13 5
Solution: (i) Since, every positive rational number is greater than every negative rational number,
18 248
therefore, > .
31 − 315
− 8 − 8
(ii) Since every negative rational number is less than 0, therefore, < 0 or 0 > .
13 13
4
(iii) Since every positive rational number is greater than 0, therefore, > 0.
5

2 8 − 14
Example 4: Arrange the rational numbers , and in descending order.
− 3 9 15
Solution: First, express the given rational numbers with positive denominators.
2 2 ×−( 1) − 2
= = . Now, LCM of 3, 9 and 15 is 45.
3
− 3 −× −( 1) 3
Expressing, each rational number with the LCM as common denominator, we get
− 2 −×215 − 30 8 85× 40 −14 −14 3 − 42
×
= = ; = = ; = =
×
3 315 45 9 95× 45 15 15 × 3 45
On comparing the numerators of the obtained rational numbers, we get 40 > – 30 > – 42.

Therefore, 40 > − 30 > − 42 or 8 > 2 > − 14 .
45 45 45 9 − 3 15