Page 62 - Maths Skills - 7

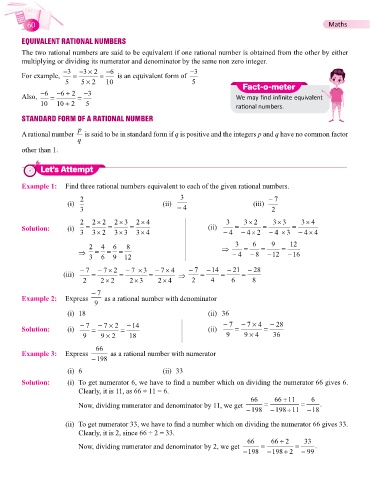
60 Maths

EQUIVALENT RATIONAL NUMBERS
The two rational numbers are said to be equivalent if one rational number is obtained from the other by either
multiplying or dividing its numerator and denominator by the same non zero integer.
−3 −×32 −6 −3
For example, = = is an equivalent form of
×
5 52 10 5 Fact-o-meter
Also, −6 = −÷6 2 = −3 We may find infinite equivalent
10 10 ÷ 2 5 rational numbers.

STANDARD FORM OF A RATIONAL NUMBER
p
A rational number is said to be in standard form if q is positive and the integers p and q have no common factor
q
other than 1.

Let’s Attempt

Example 1: Find three rational numbers equivalent to each of the given rational numbers.

2 3 − 7
(i) (ii) (iii)
3 − 4 2

2 22× 23× 24× 3 32× 33× 34×
Solution: (i) 3 = 32× = 33× = 34 (ii) − 4 = −× = − 4 × 3 = −×
44
42
×
2 4 6 8 ⇒ 3 = 6 = 9 = 12
⇒ = = = − 4 − 8 − 12 − 16
3 6 9 12

(iii) − 7 = −×72 = − 7 × 3 = −×74 ⇒ − 7 = −14 = − 21 = − 28
×
×
2 22 23 24 2 4 6 8
×
− 7
Example 2: Express as a rational number with denominator
9
(i) 18 (ii) 36

Solution: (i) − 7 = −×7 2 = −14 (ii) − 7 = −×7 4 = − 28
9 9 × 2 18 9 9 × 4 36

66
Example 3: Express as a rational number with numerator
− 198

(i) 6 (ii) 33
Solution: (i) To get numerator 6, we have to find a number which on dividing the numerator 66 gives 6.
Clearly, it is 11, as 66 ÷ 11 = 6.
66 66 11÷ 6
Now, dividing numerator and denominator by 11, we get = = .
− 198 − 198 11÷ − 18
(ii) To get numerator 33, we have to find a number which on dividing the numerator 66 gives 33.
Clearly, it is 2, since 66 ÷ 2 = 33.
66 66 2÷ 33
Now, dividing numerator and denominator by 2, we get = = .
− 198 − 198 2÷ − 99