Page 189 - Maths Skills - 7

Symmetry 187

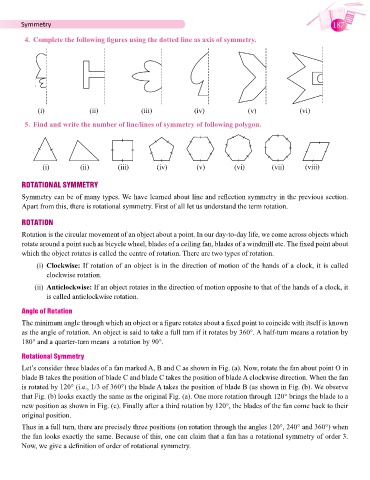
4. Complete the following figures using the dotted line as axis of symmetry.

(i) (ii) (iii) (iv) (v) (vi)

5. Find and write the number of line/lines of symmetry of following polygon.

(i) (ii) (iii) (iv) (v) (vi) (vii) (viii)

ROTATIONAL SYMMETRY

Symmetry can be of many types. We have learned about line and reflection symmetry in the previous section.
Apart from this, there is rotational symmetry. First of all let us understand the term rotation.

ROTATION
Rotation is the circular movement of an object about a point. In our day-to-day life, we come across objects which
rotate around a point such as bicycle wheel, blades of a ceiling fan, blades of a windmill etc. The fixed point about
which the object rotates is called the centre of rotation. There are two types of rotation.

(i) Clockwise: If rotation of an object is in the direction of motion of the hands of a clock, it is called
clockwise rotation.
(ii) Anticlockwise: If an object rotates in the direction of motion opposite to that of the hands of a clock, it
is called anticlockwise rotation.

Angle of Rotation

The minimum angle through which an object or a figure rotates about a fixed point to coincide with itself is known
as the angle of rotation. An object is said to take a full turn if it rotates by 360°. A half-turn means a rotation by
180° and a quarter-turn means a rotation by 90°.
Rotational Symmetry

Let’s consider three blades of a fan marked A, B and C as shown in Fig. (a). Now, rotate the fan about point O in
blade B takes the position of blade C and blade C takes the position of blade A clockwise direction. When the fan
is rotated by 120° (i.e., 1/3 of 360°) the blade A takes the position of blade B (as shown in Fig. (b). We observe
that Fig. (b) looks exactly the same as the original Fig. (a). One more rotation through 120° brings the blade to a
new position as shown in Fig. (c). Finally after a third rotation by 120°, the blades of the fan come back to their
original position.
Thus in a full turn, there are precisely three positions (on rotation through the angles 120°, 240° and 360°) when
the fan looks exactly the same. Because of this, one can claim that a fan has a rotational symmetry of order 3.
Now, we give a definition of order of rotational symmetry.