Page 30 - Maths Skill - 6

28 Maths

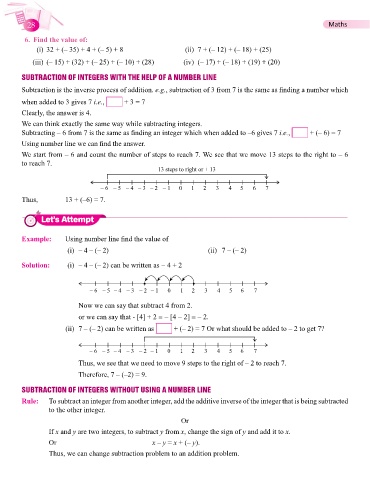
6. Find the value of:
(i) 32 + (– 35) + 4 + (– 5) + 8 (ii) 7 + (– 12) + (– 18) + (25)

(iii) (– 15) + (32) + (– 25) + (– 10) + (28) (iv) (– 17) + (– 18) + (19) + (20)

SUBTRACTION OF INTEGERS WITH THE HELP OF A NUMBER LINE

Subtraction is the inverse process of addition. e.g., subtraction of 3 from 7 is the same as finding a number which
when added to 3 gives 7 i.e., + 3 = 7
Clearly, the answer is 4.
We can think exactly the same way while subtracting integers.
Subtracting – 6 from 7 is the same as finding an integer which when added to –6 gives 7 i.e., + (– 6) = 7
Using number line we can find the answer.

We start from – 6 and count the number of steps to reach 7. We see that we move 13 steps to the right to – 6
to reach 7.
13 steps to right or + 13

– 6 – 5 – 4 – 3 – 2 – 1 0 1 2 3 4 5 6 7
Thus, 13 + (–6) = 7.

Let’s Attempt

Example: Using number line find the value of
(i) – 4 – (– 2) (ii) 7 – (– 2)

Solution: (i) – 4 – (– 2) can be written as - 4 + 2

– 6 – 5 – 4 – 3 – 2 – 1 0 1 2 3 4 5 6 7

Now we can say that subtract 4 from 2.
or we can say that - [4] + 2 = - [4 - 2] = - 2.

(ii) 7 – (– 2) can be written as + (– 2) = 7 Or what should be added to – 2 to get 7?

– 6 – 5 – 4 – 3 – 2 – 1 0 1 2 3 4 5 6 7
Thus, we see that we need to move 9 steps to the right of – 2 to reach 7.
Therefore, 7 – (–2) = 9.

SUBTRACTION OF INTEGERS WITHOUT USING A NUMBER LINE

Rule: To subtract an integer from another integer, add the additive inverse of the integer that is being subtracted
to the other integer.
Or
If x and y are two integers, to subtract y from x, change the sign of y and add it to x.
O r x – y = x + (– y).
Thus, we can change subtraction problem to an addition problem.