Page 27 - Maths Skill - 6

The Other Side of Zero 25

9. With the help of number line, give two possible integral values of x if:

(i) x > 4 (ii) x < 1 (iii) x > – 3 (iv) – 6 < x < – 3
10. Find the value of the following.
(i) | – 7 | + | – 2 | (ii) | 0 | – | 3 | (iii) | – 4 | – | 0 |

(iv) | – 5 | – | – 5 | (v) | 13 | – | – 7 | (vi) | – 9 | + | 9 |
11. Find the absolute value of each of the following.

(i) – 11 (ii) 0 (iii) 5 (iv) – 7
(v) 8 (vi) – 2 (vii) 10 (viii) – 5
12. Write the next three integers in each of the following sequences.

(i) – 20, – 15, – 10, – 5, ______ ______ ______ (ii) 12, 10, 8, 6, ______ ______ ______
(iii) – 3, – 8, – 13, – 18, ______ ______ ______ (iv) 19, 15, 11, 7, ______ ______ ______

ADDITION OF INTEGERS WITH THE HELP OF A NUMBER LINE
Steps for addition of integers on number line:

Step 1: On the number line mark one of the given integers.
Step 2: Move as many steps as the second integer to the
(i) right of the first, if the second integer is positive.

(ii) left of the first, if the second integer is negative.
Step 3: The point, thus, we reach represents the sum of the two given integers.

Let’s Attempt

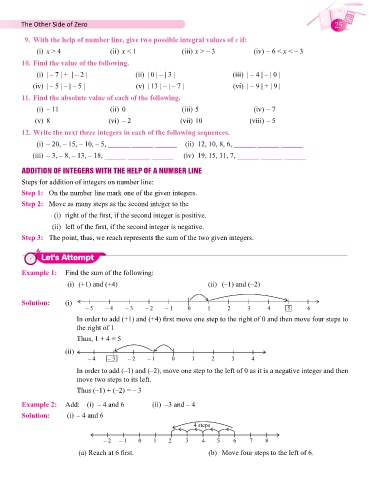
Example 1: Find the sum of the following:

(i) (+1) and (+4) (ii) (–1) and (–2)

Solution: (i)
– 5 – 4 – 3 – 2 – 1 0 1 2 3 4 5 6
In order to add (+1) and (+4) first move one step to the right of 0 and then move four steps to
the right of 1

Thus, 1 + 4 = 5
(ii)
– 4 – 3 – 2 – 1 0 1 2 3 4
In order to add (–1) and (–2), move one step to the left of 0 as it is a negative integer and then
move two steps to its left.
Thus (–1) + (–2) = – 3

Example 2: Add: (i) – 4 and 6 (ii) –3 and – 4

Solution: (i) – 4 and 6
4 steps

– 2 – 1 0 1 2 3 4 5 6 7 8
(a) Reach at 6 first. (b) Move four steps to the left of 6.