Page 190 - Math Skill - 5

188 Maths

(b) 36, 45, 55, 66, 78, ______, ______, ______.

(c) 49, 64, 81, ______, ______, ______.
2. Find the square numbers lying between 75 and 225.

3. Find the square numbers just before and after 1849. [Hint: 43 × 43 = 1849]
4. Create a triangular pattern with the following.

(a) 15 (b) 45

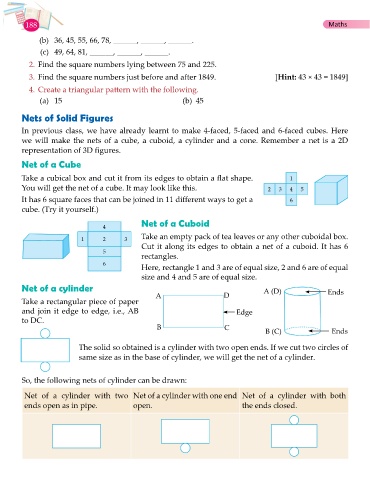
Nets of Solid Figures

In previous class, we have already learnt to make 4-faced, 5-faced and 6-faced cubes. Here
we will make the nets of a cube, a cuboid, a cylinder and a cone. Remember a net is a 2D
representation of 3D figures.

Net of a Cube

Take a cubical box and cut it from its edges to obtain a flat shape. 1
You will get the net of a cube. It may look like this. 2 3 4 5
It has 6 square faces that can be joined in 11 different ways to get a 6
cube. (Try it yourself.)

Net of a Cuboid
4
1 2 3 Take an empty pack of tea leaves or any other cuboidal box.
Cut it along its edges to obtain a net of a cuboid. It has 6
5
rectangles.
6
Here, rectangle 1 and 3 are of equal size, 2 and 6 are of equal
size and 4 and 5 are of equal size.
Net of a cylinder A (D) Ends

Take a rectangular piece of paper A D
and join it edge to edge, i.e., AB Edge
to DC.
B C B (C) Ends

The solid so obtained is a cylinder with two open ends. If we cut two circles of
same size as in the base of cylinder, we will get the net of a cylinder.

So, the following nets of cylinder can be drawn:

Net of a cylinder with two Net of a cylinder with one end Net of a cylinder with both
ends open as in pipe. open. the ends closed.