Page 22 - Mathematics Class - IX

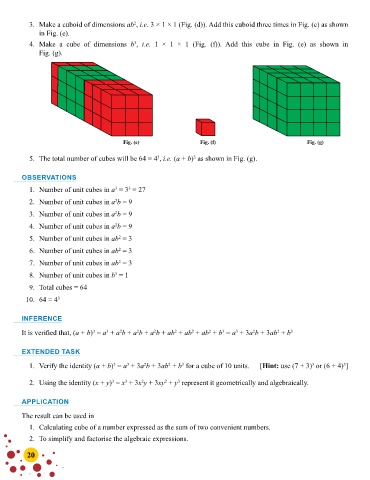
3. Make a cuboid of dimensions ab , i.e. 3 × 1 × 1 (Fig. (d)). Add this cuboid three times in Fig. (c) as shown
2
in Fig. (e).
4. Make a cube of dimensions b , i.e. 1 × 1 × 1 (Fig. (f)). Add this cube in Fig. (e) as shown in
3
Fig. (g).

Fig. (e) Fig. (f) Fig. (g)

5. The total number of cubes will be 64 = 4 , i.e. (a + b) as shown in Fig. (g).
3
3
OBSERVATIONS
1. Number of unit cubes in a = 3 = 27
3
3
2. Number of unit cubes in a b = 9
2
3. Number of unit cubes in a b = 9
2
4. Number of unit cubes in a b = 9
2
5. Number of unit cubes in ab = 3
2
6. Number of unit cubes in ab = 3
2
7. Number of unit cubes in ab = 3
2
8. Number of unit cubes in b = 1
3
9. Total cubes = 64

10. 64 = 4 3

INFERENCE

It is verified that, (a + b) = a + a b + a b + a b + ab + ab + ab + b = a + 3a b + 3ab + b 3
2
3
2
2
3
2
2
2
2
3
2
3
EXTENDED TASK
1. Verify the identity (a + b) = a + 3a b + 3ab + b for a cube of 10 units. [Hint: use (7 + 3) or (6 + 4) ]
3
3
3
2
3
2
3
2. Using the identity (x + y) = x + 3x y + 3xy + y represent it geometrically and algebraically.
3
2
2
3
3
APPLICATION
The result can be used in
1. Calculating cube of a number expressed as the sum of two convenient numbers.
2. To simplify and factorise the algebraic expressions.
20