Page 19 - Mathematics Class - IX

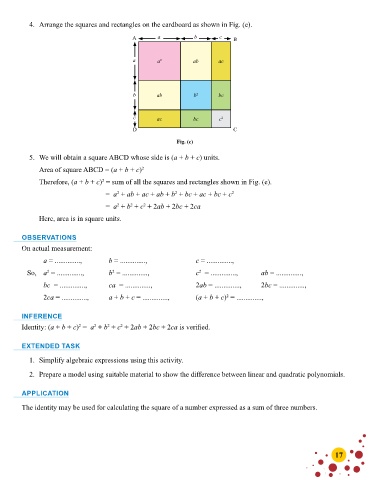
4. Arrange the squares and rectangles on the cardboard as shown in Fig. (e).

A a b c B

a a 2 ab ac

b ab b 2 bc

c ac bc c 2
D C
Fig. (e)

5. We will obtain a square ABCD whose side is (a + b + c) units.
Area of square ABCD = (a + b + c) 2
Therefore, (a + b + c) = sum of all the squares and rectangles shown in Fig. (e).
2
= a + ab + ac + ab + b + bc + ac + bc + c 2
2
2
= a + b + c + 2ab + 2bc + 2ca
2
2
2
Here, area is in square units.
OBSERVATIONS
On actual measurement:

a = .............., b = .............., c = ..............,
So, a = .............., b = .............., c = .............., ab = ..............,
2
2
2
bc = .............., ca = .............., 2ab = .............., 2bc = ..............,
2ca = .............., a + b + c = .............., (a + b + c) = ..............,
2

INFERENCE
Identity: (a + b + c) = a + b + c + 2ab + 2bc + 2ca is verified.
2
2
2
2
EXTENDED TASK
1. Simplify algebraic expressions using this activity.

2. Prepare a model using suitable material to show the difference between linear and quadratic polynomials.

APPLICATION

The identity may be used for calculating the square of a number expressed as a sum of three numbers.

17