Page 18 - Mathematics Class - IX

Unit 2 Algebra
Algebra

ACTIVITY 2.1

OBJECTIVE
To verify the algebraic identity: (a + b + c) = a + b + c + 2ab + 2bc + 2ca
2
2
2
2
MATERIALS REQUIRED
Cardboard Coloured papers Scissors
Adhesive A white paper Geometry box

PRE-REQUISITE KNOWLEDGE
1. Knowledge of polynomials 3. Concept of rectangle and its area
2. Concept of square and its area

THEORY
1. Square: A quadrilateral whose all sides are equal and all the angles are 90°.
Area of a square = (Side) 2

2. Rectangle: A quadrilateral whose opposite sides are equal and all angles are 90°.
Area of a rectangle = Length × Breadth
PROCEDURE
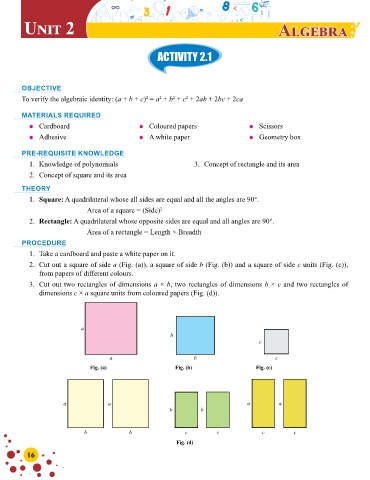
1. Take a cardboard and paste a white paper on it.

2. Cut out a square of side a (Fig. (a)), a square of side b (Fig. (b)) and a square of side c units (Fig. (c)),
from papers of different colours.

3. Cut out two rectangles of dimensions a × b, two rectangles of dimensions b × c and two rectangles of
dimensions c × a square units from coloured papers (Fig. (d)).

a
b
c

a b c
Fig. (a) Fig. (b) Fig. (c)

a a a a
b b

b b c c c c
Fig. (d)

16