Page 76 - Mathematics Class - XII

Unit IV Vect
Vectors and ors and

Three-Dimensional geometry geometry
Three-Dimensional

TOPIC - 6: Vector Algebra

ACTIVITY 6.1

OBJECTIVE
      

a c
To verify geometrically that a b c
a b
MATERIAL REQUIRED

Y Geometry box Y White paper Y Cutter Y Adhesive
Y Cardboard Y Sketch pen Y Cellotape

PRE-REQUISITE KNOWLEDGE
1. Knowledge of the concept of projection of a vector.
2. Knowledge of the concept of dot product and cross product of two vectors.

PROCEDURE
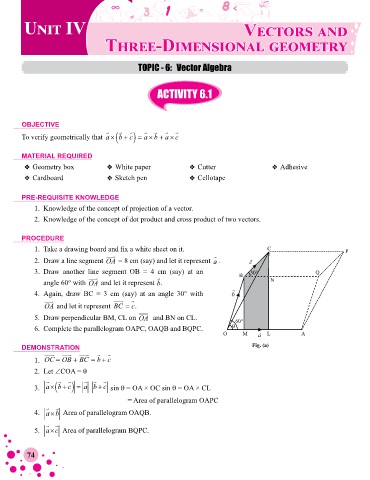
1. Take a drawing board and fix a white sheet on it.

2. Draw a line segment OA = 8 cm (say) and let it represent a .
3. Draw another line segment OB = 4 cm (say) at an


angle 60° with OA and let it represent b.
4. Again, draw BC = 3 cm (say) at an angle 30° with

OA and let it represent BC = c.

5. Draw perpendicular BM, CL on OA and BN on CL.
60°
6. Complete the parallelogram OAPC, OAQB and BQPC.

DEMONSTRATION Fig. (a)

bc
1. OC OB BC
2. Let ∠COA = q
    
3. a b c a bc sin q = OA × OC sin q = OA × CL

= Area of parallelogram OAPC
4. 
ab× Area of parallelogram OAQB.

5. ac× Area of parallelogram BQPC.

74