Page 73 - Mathematics Class - XII

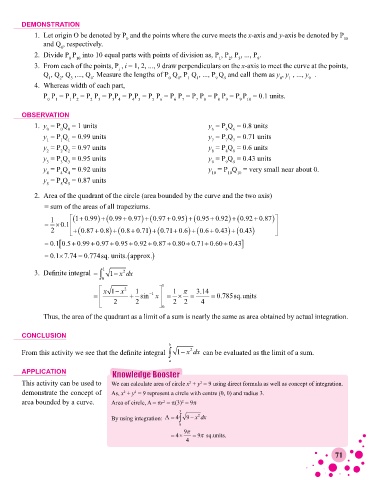
DEMONSTRATION
1. Let origin O be denoted by P and the points where the curve meets the x-axis and y-axis be denoted by P 10
0
and Q , respectively.
0
2. Divide P P into 10 equal parts with points of division as, P , P , P , ..., P .
0 10 1 2 3 9
3. From each of the points, P , i = 1, 2, ..., 9 draw perpendiculars on the x-axis to meet the curve at the points,
i
Q , Q , Q ,..., Q . Measure the lengths of P Q , P Q , ..., P Q and call them as y , y , ..., y .
1 2 3 9 0 0 1 1 9 9 0 1 9
4. Whereas width of each part,
P P = P P = P P = P P = P P = P P = P P = P P = P P = P P = 0.1 units.
0 1 1 2 2 3 3 4 4 5 5 6 6 7 7 8 8 9 9 10

OBSERVATION
1. y = P Q = 1 units y = P Q = 0.8 units
0 0 0 6 6 6
y = P Q = 0.99 units y = P Q = 0.71 units
1 1 1 7 7 7
y = P Q = 0.97 units y = P Q = 0.6 units
2 2 2 8 8 8
y = P Q = 0.95 units y = P Q = 0.43 units
3 3 3 9 9 9
y = P Q = 0.92 units y = P Q = very small near about 0.
4 4 4 10 10 10
y = P Q = 0.87 units
5 5 5
2. Area of the quadrant of the circle (area bounded by the curve and the two axis)

= sum of the areas of all trapeziums.
10 0990 97. . 0970 95. . 0950 92. . 0920. ..87
99.
1
01.

2 . 0870 .8 . 0 80 .71 . 0710 .6 . 0 60 .43 . 043
01 05 0990 97 0950 92 0870 80 0710 60 0. . . . . . . . . . .443
. . .
01 7740 774sq. units.approx.
3. Definite integral 0 1 1 xdx
2
x 1 x 2 1 1 1 314
.
sin 1 x 0 785sq.u. n nits
2 2 2 2 4
0
Thus, the area of the quadrant as a limit of a sum is nearly the same as area obtained by actual integration.

CONCLUSION
b
From this activity we see that the definite integral 1 xdx can be evaluated as the limit of a sum.
2
a
APPLICATION Knowledge Booster
This activity can be used to We can calculate area of circle x + y = 9 using direct formula as well as concept of integration.
2
2
demonstrate the concept of As, x + y = 9 represent a circle with centre (0, 0) and radius 3.
2
2
area bounded by a curve. Area of circle, A = pr = p(3) = 9p
2
2
3
2
By using integration: A 4 9 xdx
0
9
4 9 sq.units.
4
71