Page 66 - Mathematics Class - XII

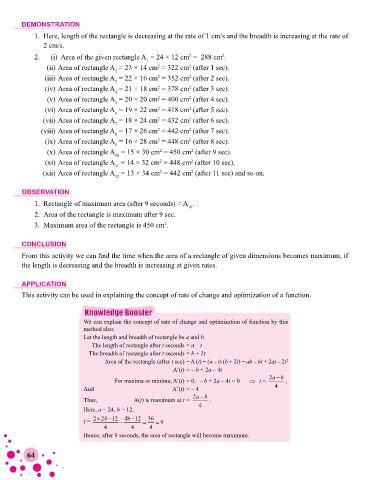
DEMONSTRATION
1. Here, length of the rectangle is decreasing at the rate of 1 cm/s and the breadth is increasing at the rate of
2 cm/s.

2. (i) Area of the given rectangle A = 24 × 12 cm = 288 cm .
2
2
1
(ii) Area of rectangle A = 23 × 14 cm = 322 cm (after 1 sec).
2
2
2
(iii) Area of rectangle A = 22 × 16 cm = 352 cm (after 2 sec).
2
2
3
(iv) Area of rectangle A = 21 × 18 cm = 378 cm (after 3 sec).
2
2
4
(v) Area of rectangle A = 20 × 20 cm = 400 cm (after 4 sec).
2
2
5
(vi) Area of rectangle A = 19 × 22 cm = 418 cm (after 5 sec).
2
2
6
(vii) Area of rectangle A = 18 × 24 cm = 432 cm (after 6 sec).
2
2
7
(viii) Area of rectangle A = 17 × 26 cm = 442 cm (after 7 sec).
2
2
8
(ix) Area of rectangle A = 16 × 28 cm = 448 cm (after 8 sec).
2
2
9
(x) Area of rectangle A = 15 × 30 cm = 450 cm (after 9 sec).
2
2
10
(xi) Area of rectangle A = 14 × 32 cm = 448 cm (after 10 sec).
2
2
11
(xii) Area of rectangle A = 13 × 34 cm = 442 cm (after 11 sec) and so on.
2
2
12
OBSERVATION
1. Rectangle of maximum area (after 9 seconds) = A .
10
2. Area of the rectangle is maximum after 9 sec.
3. Maximum area of the rectangle is 450 cm .
2
CONCLUSION
From this activity we can find the time when the area of a rectangle of given dimensions becomes maximum, if
the length is decreasing and the breadth is increasing at given rates.
APPLICATION
This activity can be used in explaining the concept of rate of change and optimization of a function.
Knowledge Booster
We can explain the concept of rate of change and optimization of function by this
method also:
Let the length and breadth of rectangle be a and b.
The length of rectangle after t seconds = a – t
The breadth of rectangle after t seconds = b + 2t
Area of the rectangle (after t sec) = A (t) = (a – t) (b + 2t) = ab – bt + 2at – 2t 2
A′(t) = – b + 2a – 4t
For maxima or minima, A′(t) = 0, – b + 2a – 4t = 0 ⇒ t = 2ab− ,
And A˝(t) = – 4 4

Thus, A(t) is maximum at t = 2ab− .
4
Here, a = 24, b = 12.
t = 22412 48 12 36 9
4 4 4
Hence, after 9 seconds, the area of rectangle will become maximum.

64