Page 63 - Mathematics Class - XII

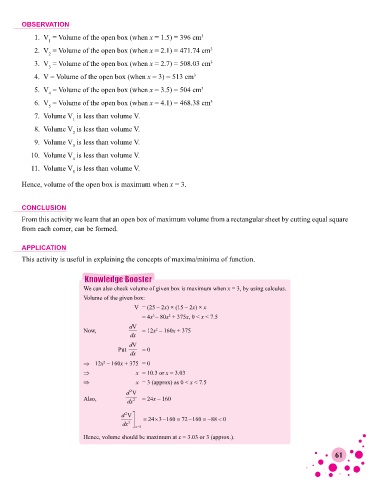
OBSERVATION

1. V = Volume of the open box (when x = 1.5) = 396 cm 3
1
2. V = Volume of the open box (when x = 2.1) = 471.74 cm 3
2
3. V = Volume of the open box (when x = 2.7) = 508.03 cm 3
3
4. V = Volume of the open box (when x = 3) = 513 cm 3

5. V = Volume of the open box (when x = 3.5) = 504 cm 3
4
6. V = Volume of the open box (when x = 4.1) = 468.38 cm 3
5
7. Volume V is less than volume V.
1
8. Volume V is less than volume V.
2
9. Volume V is less than volume V.
3
10. Volume V is less than volume V.
4
11. Volume V is less than volume V.
5
Hence, volume of the open box is maximum when x = 3.

CONCLUSION
From this activity we learn that an open box of maximum volume from a rectangular sheet by cutting equal square
from each corner, can be formed.

APPLICATION
This activity is useful in explaining the concepts of maxima/minima of function.

Knowledge Booster

We can also check volume of given box is maximum when x = 3, by using calculus.
Volume of the given box:
V = (25 – 2x) × (15 – 2x) × x
= 4x – 80x + 375x, 0 < x < 7.5
3
2
dV
Now, = 12x – 160x + 375
2
dx
dV
Put = 0
dx
⇒ 12x – 160x + 375 = 0
2
⇒ x = 10.3 or x = 3.03
⇒ x = 3 (approx) as 0 < x < 7.5
2
d V
Also, dx 2 = 24x – 160

d V 24 3 160 72 160 88 0
2
dx 2

x 3
Hence, volume should be maximum at x = 3.03 or 3 (approx.).

61