Page 61 - Mathematics Class - XII

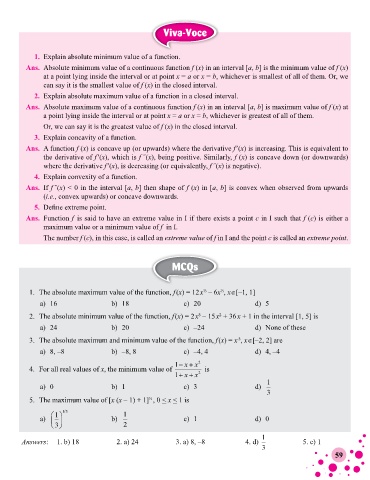
Viva-Voce

1. Explain absolute minimum value of a function.
Ans. Absolute minimum value of a continuous function f (x) in an interval [a, b] is the minimum value of f (x)
at a point lying inside the interval or at point x = a or x = b, whichever is smallest of all of them. Or, we
can say it is the smallest value of f (x) in the closed interval.

2. Explain absolute maximum value of a function in a closed interval.
Ans. Absolute maximum value of a continuous function f (x) in an interval [a, b] is maximum value of f (x) at
a point lying inside the interval or at point x = a or x = b, whichever is greatest of all of them.
Or, we can say it is the greatest value of f (x) in the closed interval.
3. Explain concavity of a function.

Ans. A function f (x) is concave up (or upwards) where the derivative f ′(x) is increasing. This is equivalent to
the derivative of f ′(x), which is f ˝(x), being positive. Similarly, f (x) is concave down (or downwards)
where the derivative f ′(x), is decreasing (or equivalently, f ˝(x) is negative).
4. Explain convexity of a function.

Ans. If f ˝(x) < 0 in the interval [a, b] then shape of f (x) in [a, b] is convex when observed from upwards
(i.e., convex upwards) or concave downwards.

5. Define extreme point.
Ans. Function f is said to have an extreme value in I if there exists a point c in I such that f (c) is either a
maximum value or a minimum value of f in I.
The number f (c), in this case, is called an extreme value of f in I and the point c is called an extreme point.

MCQs

1. The absolute maximum value of the function, f (x) = 12 x – 6x , x∈[–1, 1]
1/3
4/3
a) 16 b) 18 c) 20 d) 5
2. The absolute minimum value of the function, f (x) = 2 x3 – 15 x2 + 36 x + 1 in the interval [1, 5] is
a) 24 b) 20 c) –24 d) None of these

3. The absolute maximum and minimum value of the function, f (x) = x 3, x∈[–2, 2] are
a) 8, –8 b) –8, 8 c) –4, 4 d) 4, –4

xx
1 2
4. For all real values of x, the minimum value of is
1 xx 2
a) 0 b) 1 c) 3 d) 1
3
5. The maximum value of [x (x – 1) + 1] , 0 < x < 1 is
1/3

a) 1 13/ b) 1 c) 1 d) 0

3 2

1
Answers: 1. b) 18 2. a) 24 3. a) 8, –8 4. d) 5. c) 1
3
59