Page 16 - Mathematics Class - XII

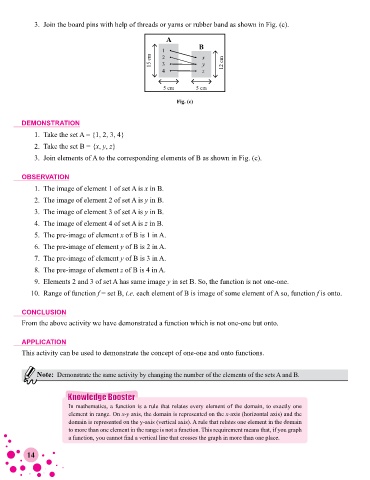
3. Join the board pins with help of threads or yarns or rubber band as shown in Fig. (c).

A
B
1 •
15 cm 2 • • x 12 cm
3 •
• y
4 • • z

5 cm 5 cm

Fig. (c)

DEMONSTRATION

1. Take the set A = {1, 2, 3, 4}
2. Take the set B = {x, y, z}

3. Join elements of A to the corresponding elements of B as shown in Fig. (c).

OBSERVATION
1. The image of element 1 of set A is x in B.
2. The image of element 2 of set A is y in B.

3. The image of element 3 of set A is y in B.
4. The image of element 4 of set A is z in B.
5. The pre-image of element x of B is 1 in A.

6. The pre-image of element y of B is 2 in A.
7. The pre-image of element y of B is 3 in A.

8. The pre-image of element z of B is 4 in A.
9. Elements 2 and 3 of set A has same image y in set B. So, the function is not one-one.
10. Range of function f = set B, i.e. each element of B is image of some element of A so, function f is onto.

CONCLUSION
From the above activity we have demonstrated a function which is not one-one but onto.

APPLICATION
This activity can be used to demonstrate the concept of one-one and onto functions.

Note: Demonstrate the same activity by changing the number of the elements of the sets A and B.

Knowledge Booster

In mathematics, a function is a rule that relates every element of the domain, to exactly one
element in range. On x-y axis, the domain is represented on the x-axis (horizontal axis) and the
domain is represented on the y-axis (vertical axis). A rule that relates one element in the domain
to more than one element in the range is not a function. This requirement means that, if you graph
a function, you cannot find a vertical line that crosses the graph in more than one place.

14