Page 68 - Mathematics Class - XI

Unit III Coordina
Coordinate Geometryte Geometry

TOPIC - 9: Straight Lines

ACTIVITY 9.1

OBJECTIVE
To verify that the equation of a line passing through the point of intersection of two lines a x + b y + c = 0 and
1
1
1
a x + b y + c = 0 is of the form (a x + b y + c ) + l (a x + b y + c ) = 0.
2 2 2 1 1 1 2 2 2
MATERIAL REQUIRED
 Cardboard  Graph paper

 Pencil  Glue
 White chart paper  Ruler

PRE-REQUISITE KNOWLEDGE
1. Knowledge of general equation of a line

2. Knowledge of plotting the graph of the equation of a line

PROCEDURE
1. Take a cardboard of convenient size and paste a
white chart paper on it.
2. Paste a graph paper on the white chart paper.

3. Draw two perpendicular lines X′OX and YOY′
on the graph paper. Take same scale for marking
points on x and y-axes.

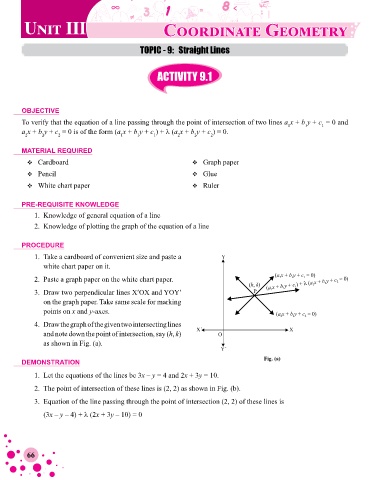
4. Draw the graph of the given two intersecting lines
and note down the point of intersection, say (h, k)
as shown in Fig. (a).

DEMONSTRATION Fig. (a)
1. Let the equations of the lines be 3x – y = 4 and 2x + 3y = 10.

2. The point of intersection of these lines is (2, 2) as shown in Fig. (b).

3. Equation of the line passing through the point of intersection (2, 2) of these lines is
(3x – y – 4) + l (2x + 3y – 10) = 0

66