Page 50 - Mathematics Class - XI

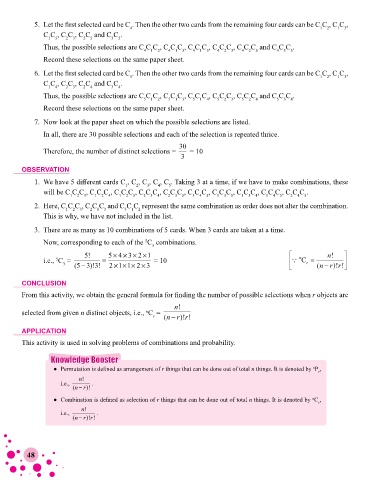
5. Let the first selected card be C . Then the other two cards from the remaining four cards can be C C , C C ,
4 1 2 1 3
C C , C C , C C and C C .
1 5 2 3 2 5 3 5
Thus, the possible selections are C C C , C C C , C C C , C C C , C C C and C C C .
4
3
4
5
1
1
4
2
1
4
2
4
3
5
5
3
4
2
Record these selections on the same paper sheet.
6. Let the first selected card be C . Then the other two cards from the remaining four cards can be C C , C C ,
3
5
1
2
1
C C , C C , C C and C C .
2
3
4
4
3
2
4
1
Thus, the possible selections are C C C , C C C , C C C , C C C , C C C and C C C .
5 1 2 5 1 3 5 1 4 5 2 3 5 2 4 5 3 4
Record these selections on the same paper sheet.
7. Now look at the paper sheet on which the possible selections are listed.
In all, there are 30 possible selections and each of the selection is repeated thrice.
Therefore, the number of distinct selections = 30 = 10
3
OBSERVATION
1. We have 5 different cards C , C , C , C , C . Taking 3 at a time, if we have to make combinations, these
2
1
5
4
3
will be C C C , C C C , C C C , C C C , C C C , C C C , C C C , C C C , C C C , C C C .
1 2 3 1 2 4 1 2 5 2 3 4 2 3 5 3 4 5 1 3 5 1 3 4 1 4 5 2 4 5
2. Here, C C C , C C C and C C C represent the same combination as order does not alter the combination.
1
3
1
2
3
2
2
1
3
This is why, we have not included in the list.
3. There are as many as 10 combinations of 5 cards. When 3 cards are taken at a time.
Now, corresponding to each of the C combinations.
5
3
n
i.e., C = 5! = 5 432 1×× ×× = 10   C = n! 
5


nr r
××× ×
3 ( 53 3)!!− 21 12 3  r ( − )!! 
CONCLUSION
From this activity, we obtain the general formula for finding the number of possible selections when r objects are
n!
selected from given n distinct objects, i.e., C =
n
r ( nr r)!!−
APPLICATION
This activity is used in solving problems of combinations and probability.
Knowledge Booster
● Permutation is defined as arrangement of r things that can be done out of total n things. It is denoted by P ,
n
r
i.e., n! .
( nr)!−
● Combination is defined as selection of r things that can be done out of total n things. It is denoted by C ,
n
r
i.e., n! .
( − )!!
nr r
48