Page 39 - Mathematics Class - XI

DEMONSTRATION
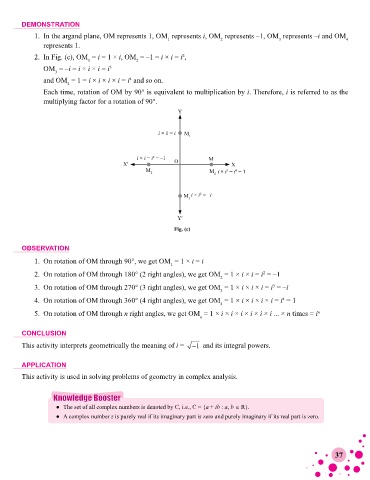
1. In the argand plane, OM represents 1, OM represents i, OM represents –1, OM represents –i and OM
4
3
2
1
represents 1.
2. In Fig. (c), OM = i = 1 × i, OM = –1 = i × i = i ,
2
1 2
OM = –i = i × i × i = i 3
3
and OM = 1 = i × i × i × i = i and so on.
4
4
Each time, rotation of OM by 90° is equivalent to multiplication by i. Therefore, i is referred to as the
multiplying factor for a rotation of 90°.
Y

i × 1 = i M
1

i × i = i = –1 M
2
X′ O X
M 2 M 4 i × i = i = 1
4
3

2
M i × i = –i
3
Y′
Fig. (c)

OBSERVATION

1. On rotation of OM through 90°, we get OM = 1 × i = i
1
2. On rotation of OM through 180° (2 right angles), we get OM = 1 × i × i = i = –1
2
2
3. On rotation of OM through 270° (3 right angles), we get OM = 1 × i × i × i = i = –i
3
3
4. On rotation of OM through 360° (4 right angles), we get OM = 1 × i × i × i × i = i = 1
4
4
5. On rotation of OM through n right angles, we get OM = 1 × i × i × i × i × i × i ... × n times = i n
n
CONCLUSION
This activity interprets geometrically the meaning of i = −1 and its integral powers.

APPLICATION

This activity is used in solving problems of geometry in complex analysis.

Knowledge Booster
● The set of all complex numbers is denoted by C, i.e., C = {a + ib : a, b ∈ R}.
● A complex number z is purely real if its imaginary part is zero and purely imaginary if its real part is zero.

37