Page 58 - Mathematics Class - X

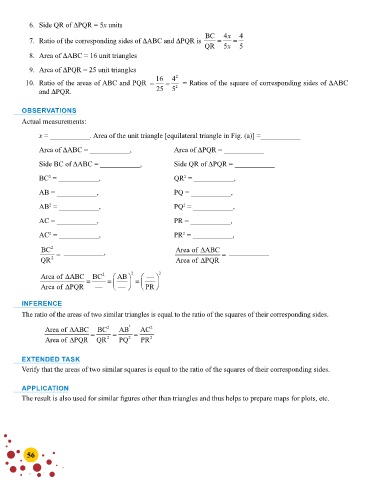
6. Side QR of ∆PQR = 5x units

7. Ratio of the corresponding sides of ∆ABC and ∆PQR is BC = 4x = 4
QR 5x 5
8. Area of ∆ABC = 16 unit triangles

9. Area of ∆PQR = 25 unit triangles
16 4 2
10. Ratio of the areas of ABC and PQR = = 2 = Ratios of the square of corresponding sides of ∆ABC
and ∆PQR. 25 5

OBSERVATIONS
Actual measurements:

x = ___________. Area of the unit triangle [equilateral triangle in Fig. (a)] =___________

Area of ∆ABC = ___________, Area of ∆PQR = ___________

Side BC of ∆ABC = ___________, Side QR of ∆PQR = ___________

BC = ___________, QR = ___________,
2
2
AB = ___________, PQ = ___________,

AB 2 = ___________, PQ = ___________,
2
AC = ___________, PR = ___________,

AC 2 = ___________, PR = ___________,
2
BC 2 = ___________, Area of ABC∆ = ___________

QR 2 Area of PQR
∆
Area of ABC∆ = BC 2 =  AB  2 =  —  2
Area of PQR —   —     PR  
∆

INFERENCE
The ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding sides.

Area of ABC∆ BC 2 AB 2 AC 2
= = =
Area of PQR QR 2 PQ 2 PR 2
∆

EXTENDED TASK
Verify that the areas of two similar squares is equal to the ratio of the squares of their corresponding sides.

APPLICATION
The result is also used for similar figures other than triangles and thus helps to prepare maps for plots, etc.

56