Page 57 - Mathematics Class - X

ACTIVITY 4.3

OBJECTIVE
To verify experimentally that the ratio of the areas of two similar triangles is equal to the ratio of the squares of
their corresponding sides.

MATERIALS REQUIRED

Coloured papers Sketch pen Glue
Geometry box White paper Cardboard

PRE-REQUISITE KNOWLEDGE
1. Concept of similarity of triangles 3. Concept of congruency of triangles

2. Area of triangles 4. Theorem related to area of two similar triangles

THEORY
1. Two figures are said to be congruent, if they have the same shape and exactly same size. In the same way,
two triangles are said to be congruent, if they have same shape and same size (same area).
2. The ratio of the areas of two similar triangles is equal to the ratio of square of their corresponding sides.
For given ∆ABC and ∆PQR,

area of ABC∆  AB  2  BC  2  CA  2
=   =   =  
area of PQR∆  PQ   QR   RP 

PROCEDURE

1. Paste a white paper on a cardboard of suitable length.
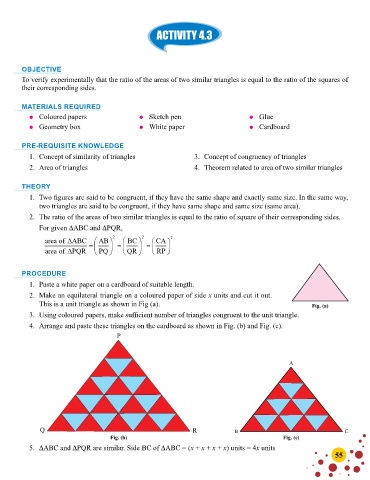
2. Make an equilateral triangle on a coloured paper of side x units and cut it out.
This is a unit triangle as shown in Fig (a). Fig. (a)
3. Using coloured papers, make sufficient number of triangles congruent to the unit triangle.
4. Arrange and paste these triangles on the cardboard as shown in Fig. (b) and Fig. (c).

Fig. (b) Fig. (c)
5. ∆ABC and ∆PQR are similar. Side BC of ∆ABC = (x + x + x + x) units = 4x units
55