Page 44 - Revised Maths Wisdom Class - 6

42 MATHS

11. The number of common prime factors of 75,60,105 are:
(a) 2 (b) 3 (c) 4 (d) 5

12. Express 44 as the sum of two odd primes.
13. Write all factors of 96.
14. Write all the numbers less than 100 which are common multiples of 4 and 6.
15. State whether the following statements are true or false. Justify your answer:

(a) Prime numbers do not have any factors. (b) The sum of three odd numbers is even.
(c) The whole number 13 lies between 11 and 12.

16. Choose three numbers to make a correct number sentence.
21, 22, 23, 24, 25, 26, 27, 28, 29

_______ + _______ + _______ = a multiple of 10.

17. Ram is thinking of a number, it is more than 30 and less than 50, it is a multiple of both 3 and 5. What number
is Ram thinking?

Prime Factorisation of a Number
We have already learnt how to find the factors of a given number. Let us take a number 72. It is a composite
number and its factors are 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36 and 72. But all these factors are not prime numbers.
When we express a given number as a product of only prime numbers it is called prime factorisation. Any
composite number can be expressed as a product of prime numbers in only one way. For example,
30 = 2 × 3 × 5 45 = 3 × 3 × 5 12 = 2 × 2 × 3
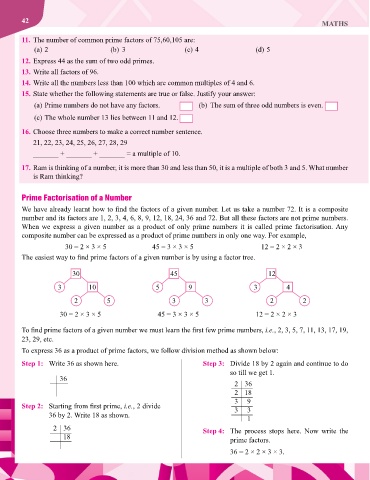
The easiest way to find prime factors of a given number is by using a factor tree.

30 45 12

3 10 5 9 3 4

2 5 3 3 2 2

30 = 2 × 3 × 5 45 = 3 × 3 × 5 12 = 2 × 2 × 3

To find prime factors of a given number we must learn the first few prime numbers, i.e., 2, 3, 5, 7, 11, 13, 17, 19,
23, 29, etc.
To express 36 as a product of prime factors, we follow division method as shown below:

Step 1: Write 36 as shown here. Step 3: Divide 18 by 2 again and continue to do
so till we get 1.
36
236
218
3 9
Step 2: Starting from first prime, i.e., 2 divide 3 3
36 by 2. Write 18 as shown. 1
236 Step 4: The process stops here. Now write the
18 prime factors.

36 = 2 × 2 × 3 × 3.