Page 40 - Revised Maths Wisdom Class - 6

38 MATHS

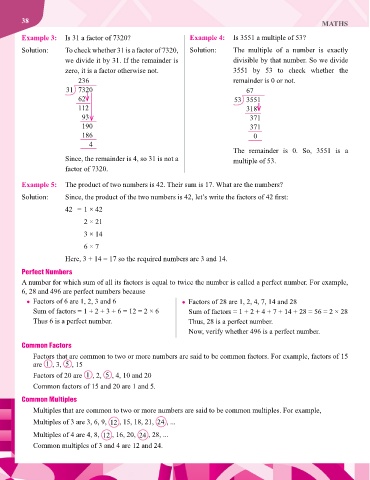
Example 3: Is 31 a factor of 7320? Example 4: Is 3551 a multiple of 53?
Solution: To check whether 31 is a factor of 7320, Solution: The multiple of a number is exactly
we divide it by 31. If the remainder is divisible by that number. So we divide

zero, it is a factor otherwise not. 3551 by 53 to check whether the
236 remainder is 0 or not.
31 7320 67
62 53 3551
112 318
93 371
190 371
186 0
4
The remainder is 0. So, 3551 is a
Since, the remainder is 4, so 31 is not a multiple of 53.
factor of 7320.

Example 5: The product of two numbers is 42. Their sum is 17. What are the numbers?

Solution: Since, the product of the two numbers is 42, let’s write the factors of 42 first:
42 = 1 × 42

2 × 21
3 × 14

6 × 7
Here, 3 + 14 = 17 so the required numbers are 3 and 14.

Perfect Numbers
A number for which sum of all its factors is equal to twice the number is called a perfect number. For example,
6, 28 and 496 are perfect numbers because
● Factors of 6 are 1, 2, 3 and 6 ● Factors of 28 are 1, 2, 4, 7, 14 and 28
Sum of factors = 1 + 2 + 3 + 6 = 12 = 2 × 6 Sum of factors = 1 + 2 + 4 + 7 + 14 + 28 = 56 = 2 × 28
Thus 6 is a perfect number. Thus, 28 is a perfect number.
Now, verify whether 496 is a perfect number.

Common Factors
Factors that are common to two or more numbers are said to be common factors. For example, factors of 15
are 1 , 3, 5 , 15
Factors of 20 are 1 , 2, 5 , 4, 10 and 20

Common factors of 15 and 20 are 1 and 5.
Common Multiples

Multiples that are common to two or more numbers are said to be common multiples. For example,
Multiples of 3 are 3, 6, 9, 12 , 15, 18, 21, 24 , ...

Multiples of 4 are 4, 8, 12 , 16, 20, 24 , 28, ...
Common multiples of 3 and 4 are 12 and 24.