Page 42 - Revised Maths Wisdom Class - 6
P. 42
40 MATHS
Remember!er!
Rememb
As 1 has only one factor hence it is neither prime number nor composite number.
2 is the smallest even prime number.
0 is neither prime number nor composite number.
Twin Primes and Co-primes
The pair of prime numbers that differ by 2 are called twin primes. For example, (3, 5), (5, 7), (11, 13),
(17, 19) are all twin primes.
Two numbers are said to be co-prime when they have only 1 as their common factor. For example, (3, 7), (16, 25)
are co-primes.
Rememb
Remember!er!
There are infinite number of pairs of twin prime numbers.
Co-primes are not always prime but pair of any primes is always co-prime.
A prime triplet is a set of three consecutive prime numbers with common difference 2. For example, (3, 5, 7) is
the only known prime triplet.
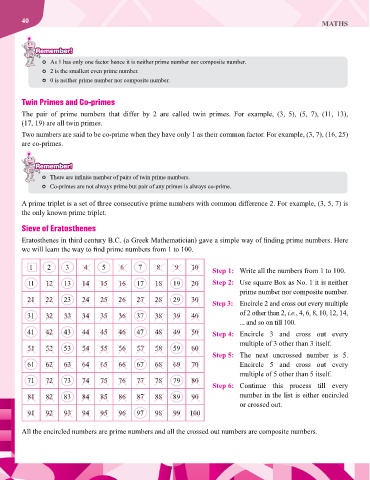
Sieve of Eratosthenes
Eratosthenes in third century B.C. (a Greek Mathematician) gave a simple way of finding prime numbers. Here
we will learn the way to find prime numbers from 1 to 100.
1 2 3 4 5 6 7 8 9 10 Step 1: Write all the numbers from 1 to 100.
11 12 13 14 15 16 17 18 19 20 Step 2: Use square Box as No. 1 it is neither
prime number nor composite number.
21 22 23 24 25 26 27 28 29 30
Step 3: Encircle 2 and cross out every multiple
31 32 33 34 35 36 37 38 39 40 of 2 other than 2, i.e., 4, 6, 8, 10, 12, 14,
... and so on till 100.
41 42 43 44 45 46 47 48 49 50 Step 4: Encircle 3 and cross out every
multiple of 3 other than 3 itself.
51 52 53 54 55 56 57 58 59 60
Step 5: The next uncrossed number is 5.
61 62 63 64 65 66 67 68 69 70 Encircle 5 and cross out every
multiple of 5 other than 5 itself.
71 72 73 74 75 76 77 78 79 80
Step 6: Continue this process till every
81 82 83 84 85 86 87 88 89 90 number in the list is either encircled
or crossed out.
91 92 93 94 95 96 97 98 99 100
All the encircled numbers are prime numbers and all the crossed out numbers are composite numbers.