Page 24 - Maths Skills - 8

22 Maths

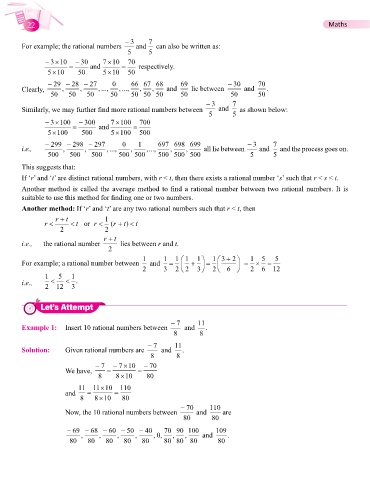
For example; the rational numbers − 3 and 7 can also be written as:
5 5
30 and 710 70 respectively.

310

510 50 510 50
− − 29 28 − 27 0 66 67 68 69 − 30 70
Clearly, , , , ..., , ..., , , and lie betweeen and .
50 50 50 50 50 50 50 50 50 50
− 3 7
Similarly, we may further find more rational numbers between and as shown below:
5 5
3 100 300 and 7 100 700
5 100 500 5 100 500

- - 299 298 - 297 0 1 697 698 6999 − 3 7
i.e., , , , ..., , ,..., , , all lie between and and the process goes on.
500 500 500 500 500 500 500 500 5 5
This suggests that:

If ‘r’ and ‘t’ are distinct rational numbers, with r < t, then there exists a rational number ‘s’ such that r < s < t.
Another method is called the average method to find a rational number between two rational numbers. It is
suitable to use this method for finding one or two numbers.
Another method: If ‘r’ and ‘t’ are any two rational numbers such that r < t, then
r t 1
r t or r ( r t ) t
2 2
r + t
i.e., the rational number lies between r and t.
2

For example; a rational number between 1 and 1 1 1 1 1 32 1 5 5

2 3 2 2 3 2 6 2 6 12
1 5 1
i.e., < < .
2 12 3
Let’s Attempt
Example 1: Insert 10 rational numbers between − 7 and 11 .
8 8

Solution: Given rational numbers are − 7 and 11 .
8 8
We have, 7 710 70

8 810 80
11 11 10 110
and
8 810 80

Now, the 10 rational numbers between - 70 and 110 are
80 80
- - 69 - 68 - 60 50 - 40 70 90 100 109
, , , , 0 ,, , , and .
80 80 80 80 80 80 80 80 80