Page 13 - Maths Skills - 8

Rational Numbers 11

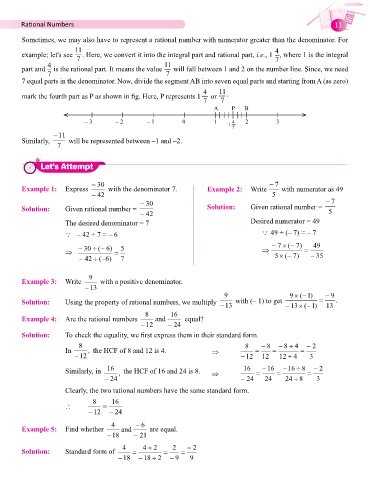
Sometimes, we may also have to represent a rational number with numerator greater than the denominator. For
11 4
example; let's see 7 . Here, we convert it into the integral part and rational part, i.e., 1 , where 1 is the integral
7
4
part and is the rational part. It means the value 11 will fall between 1 and 2 on the number line. Since, we need
7
7
7 equal parts in the denominator. Now, divide the segment AB into seven equal parts and starting from A (as zero)
4 11
mark the fourth part as P as shown in fig. Here, P represents 1 or ·
7 7
A P B
– 3 – 2 – 1 0 1 1 4 2 3
7
−11
Similarly, will be represented between –1 and –2.
7

Let’s Attempt

Example 1: Express − 30 with the denominator 7. Example 2: Write − 7 with numerator as 49
− 42 5
− 30 − 7
Solution: Given rational number = Solution: Given rational number =
− 42 5
The desired denominator = 7 Desired numerator = 49
 – 42 ÷ 7 = – 6  49 ÷ (– 7) = – 7

6)
⇒ 30 ( 5 ⇒ 7 ( 7) 49
42 6( ) 7 5 7( ) 35

Example 3: Write 9 with a positive denominator.
13−
9 9 ( 1) 9
Solution: Using the property of rational numbers, we multiply with (– 1) to get .
− 13 13 ( 1) 13

Example 4: Are the rational numbers 8 and 16 equal?
− 12 − 24
Solution: To check the equality, we first express them in their standard form.

84
In 8 , the HCF of 8 and 12 is 4. ⇒ 8 8 2
− 12 12 12 12 4 3

Similarly, in 16 , the HCF of 16 and 24 is 8. ⇒ 16 16 16 8 2
− 24 24 24 24 8 3

Clearly, the two rational numbers have the same standard form.

∴ 8 16
12 24

Example 5: Find whether 4 and − 6 are equal.
− 18 − 21

Solution: Standard form of 4 42 2 2
18 18 2 9 9