Page 12 - Maths Skills - 8

10 Maths

Important Features of Rational Numbers
I. If p is a rational number and ‘n’ is an integer where n ≠ 0, then p pn pn .
q
q qn qn

p 2 2 23 6 p pn
For example, if , n 3, then , So, and are equivalent rational numbers.

q 5 5 53 15 q qn
p p p n
II. If is a rational number and ‘n’ is an integer where n ≠ 0, then .
q q q n
p 12 12 12 3 4 p p ÷ n
For example, if , n 3, then , So, and are equivalent rational numbers.
q 21 21 21 3 7 q q ÷ n
p r p r
III. Two rational numbers and are equal if p × s = q × r i.e.,
q s q s

2 4
For example; =
5 10
2 4
Since i.e., 2 × 10 = 4 × 5 = 20
5 10

So, 2 and 4 are equivalent rational numbers.
5 10
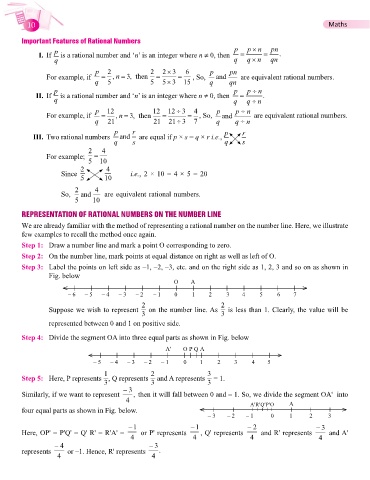
REPRESENTATION OF RATIONAL NUMBERS ON THE NUMBER LINE

We are already familiar with the method of representing a rational number on the number line. Here, we illustrate
few examples to recall the method once again.

Step 1: Draw a number line and mark a point O corresponding to zero.
Step 2: On the number line, mark points at equal distance on right as well as left of O.
Step 3: Label the points on left side as –1, –2, –3, etc. and on the right side as 1, 2, 3 and so on as shown in
Fig. below
O A

– 6 – 5 – 4 – 3 – 2 – 1 0 1 2 3 4 5 6 7
2 2
Suppose we wish to represent on the number line. As is less than 1. Clearly, the value will be
3 3
represented between 0 and 1 on positive side.
Step 4: Divide the segment OA into three equal parts as shown in Fig. below

A′ O P Q A

– 5 – 4 – 3 – 2 – 1 0 1 2 3 4 5
1 2 3
Step 5: Here, P represents , Q represents and A represents = 1.
3 3 3
Similarly, if we want to represent − 3 , then it will fall between 0 and – 1. So, we divide the segment OA' into
4 A′R′Q′P′O A
four equal parts as shown in Fig. below.
– 3 – 2 – 1 0 1 2 3

−1 −1 − 2 − 3
Here, OP' = P'Q' = Q' R' = R'A' = or P' represents , Q' represents and R' represents and A'
4 4 4 4
− 4 − 3
represents or –1. Hence, R' represents .
4 4