Page 18 - Maths Skills - 8

16 Maths

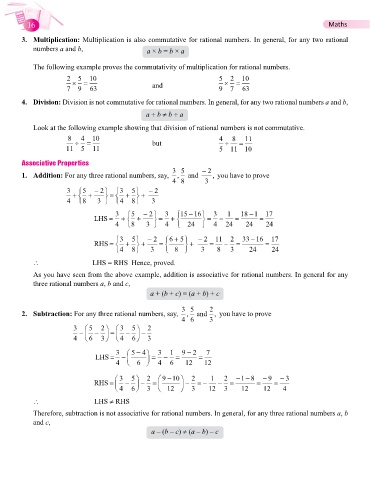
3. Multiplication: Multiplication is also commutative for rational numbers. In general, for any two rational
numbers a and b, a × b = b × a

The following example proves the commutativity of multiplication for rational numbers.

2 5 10 5 2 10
7 9 63 and 9 7 63
4. Division: Division is not commutative for rational numbers. In general, for any two rational numbers a and b,
a ÷ b ≠ b ÷ a

Look at the following example showing that division of rational numbers is not commutative.

8 4 10 but 4 8 11
11 5 11 5 11 10

Associative Properties
3 5 − 2
1. Addition: For any three rational numbers, say, , and , you have to prove
4 8 3
3 5 2 3 5 2

4 8 3 4 8 3
5
2
15
LHS = 3 +  + −  3 +  −16  3 − 1 = 18 −1 = 17
 =
 =
4 8 3  4  24  4 24 24 24
+ 
3 5  − 2 65 − 2 11 2 33 −16 17
RHS =  +  + =   + = − = =
 4 8  3  8  3 8 3 24 24
∴ LHS = RHS Hence, proved.
As you have seen from the above example, addition is associative for rational numbers. In general for any
three rational numbers a, b and c,
a + (b + c) = (a + b) + c

3 5 2
2. Subtraction: For any three rational numbers, say, , and , you have to prove
4 6 3
3 5 2 3 5 2

4 6 3 4 6 3
− 
LHS = 3 −  54  = 3 − 1 = 9 − 2 = 7

4  6  4 6 12 12

RHS 3 5 2 910 2 1 2 18 9 3

4 6 3 12 3 12 3 12 12 4
∴ LHS ≠ RHS
Therefore, subtraction is not associative for rational numbers. In general, for any three rational numbers a, b
and c,
a – (b – c) ≠ (a – b) – c