Page 159 - Maths Skills - 7

Lines and Angles 157

(iii) a pair of interior angles (or exterior angles) on the same Fact-o-meter
side of the transversal is supplementary.
Then the two lines are parallel. Lines perpendicular to the same
lines are parallel to each other.

Let’s Attempt

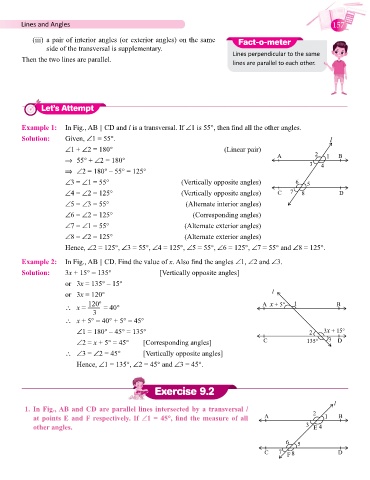
Example 1: In Fig., AB || CD and l is a transversal. If ∠1 is 55°, then find all the other angles.

Solution: Given, ∠1 = 55°. l
∠1 + ∠2 = 180° (Linear pair) 2
⇒ 55° + ∠2 = 180° A 3 1 B
⇒ ∠2 = 180° – 55° = 125° 4
∠3 = ∠1 = 55° (Vertically opposite angles) 6 5

∠4 = ∠2 = 125° (Vertically opposite angles) C 7 8 D
∠5 = ∠3 = 55° (Alternate interior angles)
∠6 = ∠2 = 125° (Corresponding angles)

∠7 = ∠1 = 55° (Alternate exterior angles)
∠8 = ∠2 = 125° (Alternate exterior angles)
Hence, ∠2 = 125°, ∠3 = 55°, ∠4 = 125°, ∠5 = 55°, ∠6 = 125°, ∠7 = 55° and ∠8 = 125°.

Example 2: In Fig., AB || CD. Find the value of x. Also find the angles ∠1, ∠2 and ∠3.
Solution: 3x + 15° = 135° [Vertically opposite angles]

or 3x = 135° – 15°
or 3x = 120° l
\ x = 120º = 40° A x + 5° 1 B
3
\ x + 5° = 40° + 5° = 45°
∠1 = 180° – 45° = 135° 2 3x + 15°

∠2 = x + 5° = 45° [Corresponding angles] C 135° 3 D
\ ∠3 = ∠2 = 45° [Vertically opposite angles]
Hence, ∠1 = 135°, ∠2 = 45° and ∠3 = 45°.

Exercise 9.2

1. In Fig., AB and CD are parallel lines intersected by a transversal l
at points E and F respectively. If ∠1 = 45°, find the measure of all
other angles.

C D