Page 158 - Maths Skills - 7

156 Maths

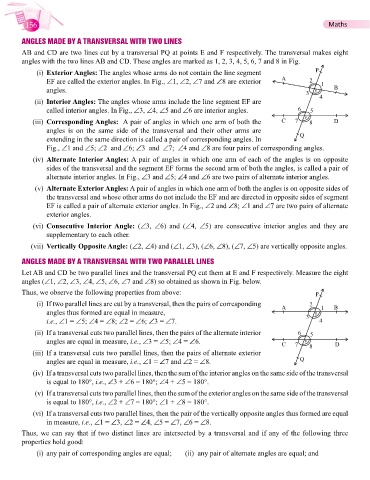
ANGLES MADE BY A TRANSVERSAL WITH TWO LINES
AB and CD are two lines cut by a transversal PQ at points E and F respectively. The transversal makes eight
angles with the two lines AB and CD. These angles are marked as 1, 2, 3, 4, 5, 6, 7 and 8 in Fig.
(i) Exterior Angles: The angles whose arms do not contain the line segment P
EF are called the exterior angles. In Fig., ∠1, ∠2, ∠7 and ∠8 are exterior A 2 1
angles. 3 E B
(ii) Interior Angles: The angles whose arms include the line segment EF are 4
called interior angles. In Fig., ∠3, ∠4, ∠5 and ∠6 are interior angles. 6 5
(iii) Corresponding Angles: A pair of angles in which one arm of both the C 7 F 8 D
angles is on the same side of the transversal and their other arms are
extending in the same direction is called a pair of corresponding angles. In Q
Fig., ∠1 and ∠5; ∠2 and ∠6; ∠3 and ∠7; ∠4 and ∠8 are four pairs of corresponding angles.
(iv) Alternate Interior Angles: A pair of angles in which one arm of each of the angles is on opposite
sides of the transversal and the segment EF forms the second arm of both the angles, is called a pair of
alternate interior angles. In Fig., ∠3 and ∠5; ∠4 and ∠6 are two pairs of alternate interior angles.
(v) Alternate Exterior Angles: A pair of angles in which one arm of both the angles is on opposite sides of
the transversal and whose other arms do not include the EF and are directed in opposite sides of segment
EF is called a pair of alternate exterior angles. In Fig., ∠2 and ∠8; ∠1 and ∠7 are two pairs of alternate
exterior angles.

(vi) Consecutive Interior Angle: (∠3, ∠6) and (∠4, ∠5) are consecutive interior angles and they are
supplementary to each other.

(vii) Vertically Opposite Angle: (∠2, ∠4) and (∠1, ∠3), (∠6, ∠8), (∠7, ∠5) are vertically opposite angles.

ANGLES MADE BY A TRANSVERSAL WITH TWO PARALLEL LINES
Let AB and CD be two parallel lines and the transversal PQ cut them at E and F respectively. Measure the eight
angles (∠1, ∠2, ∠3, ∠4, ∠5, ∠6, ∠7 and ∠8) so obtained as shown in Fig. below.
Thus, we observe the following properties from above: P

(i) If two parallel lines are cut by a transversal, then the pairs of corresponding A 2 1 B
angles thus formed are equal in measure, E
i.e., ∠1 = ∠5; ∠4 = ∠8; ∠2 = ∠6; ∠3 = ∠7. 3 4

(ii) If a transversal cuts two parallel lines, then the pairs of the alternate interior 6 5
angles are equal in measure, i.e., ∠3 = ∠5; ∠4 = ∠6. C 7 F 8 D

(iii) If a transversal cuts two parallel lines, then the pairs of alternate exterior
angles are equal in measure, i.e., ∠1 = ∠7 and ∠2 = ∠8. Q
(iv) If a transversal cuts two parallel lines, then the sum of the interior angles on the same side of the transversal
is equal to 180°, i.e., ∠3 + ∠6 = 180°; ∠4 + ∠5 = 180°.
(v) If a transversal cuts two parallel lines, then the sum of the exterior angles on the same side of the transversal
is equal to 180°, i.e., ∠2 + ∠7 = 180°; ∠1 + ∠8 = 180°.
(vi) If a transversal cuts two parallel lines, then the pair of the vertically opposite angles thus formed are equal
in measure, i.e., ∠1 = ∠3, ∠2 = ∠4, ∠5 = ∠7, ∠6 = ∠8.
Thus, we can say that if two distinct lines are intersected by a transversal and if any of the following three
properties hold good:
(i) any pair of corresponding angles are equal; (ii) any pair of alternate angles are equal; and