Page 107 - Maths Skills - 7

Exponents and Powers 105

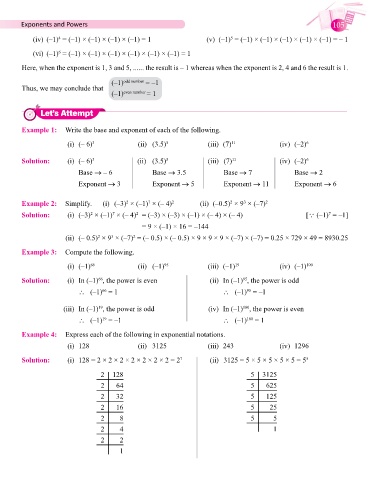
(iv) (–1) = (–1) × (–1) × (–1) × (–1) = 1 (v) (–1) = (–1) × (–1) × (–1) × (–1) × (–1) = – 1
5
4
(vi) (–1) = (–1) × (–1) × (–1) × (–1) × (–1) × (–1) = 1
6
Here, when the exponent is 1, 3 and 5, ...... the result is – 1 whereas when the exponent is 2, 4 and 6 the result is 1.

(–1) odd number = –1
Thus, we may conclude that
(–1) even number = 1

Let’s Attempt

Example 1: Write the base and exponent of each of the following.

(i) (– 6) (ii) (3.5) (iii) (7) (iv) (–2) 6
3
11
5
Solution: (i) (– 6) (ii) (3.5) 5 (iii) (7) 11 (iv) (–2) 6
3
Base → – 6 Base → 3.5 Base → 7 Base → 2
Exponent → 3 Exponent → 5 Exponent → 11 Exponent → 6

Example 2: Simplify. (i) (–3) × (–1) × (– 4) (ii) (–0.5) × 9 × (–7) 2
2
2
7
2
3
Solution: (i) (–3) × (–1) × (– 4) = (–3) × (–3) × (–1) × (– 4) × (– 4) [Q (–1) = –1]
2
7
2
7
= 9 × (–1) × 16 = –144
(ii) (– 0.5) × 9 × (–7) = (– 0.5) × (– 0.5) × 9 × 9 × 9 × (–7) × (–7) = 0.25 × 729 × 49 = 8930.25
2
2
3
Example 3: Compute the following.
(i) (–1) (ii) (–1) (iii) (–1) (iv) (–1) 100
66
95
19
Solution: (i) In (–1) , the power is even (ii) In (–1) , the power is odd
95
66
\ (–1) = 1 \ (–1) = –1
95
66
(iii) In (–1) , the power is odd (iv) In (–1) , the power is even
100
19
\ (–1) = –1 \ (–1) = 1
100
19
Example 4: Express each of the following in exponential notations.
(i) 128 (ii) 3125 (iii) 243 (iv) 1296
Solution: (i) 128 = 2 × 2 × 2 × 2 × 2 × 2 × 2 = 2 7 (ii) 3125 = 5 × 5 × 5 × 5 × 5 = 5 5
2 128 5 3125
2 64 5 625
2 32 5 125

2 16 5 25
2 8 5 5

2 4 1
2 2
1