Page 44 - Math Skill - 4

42 Maths

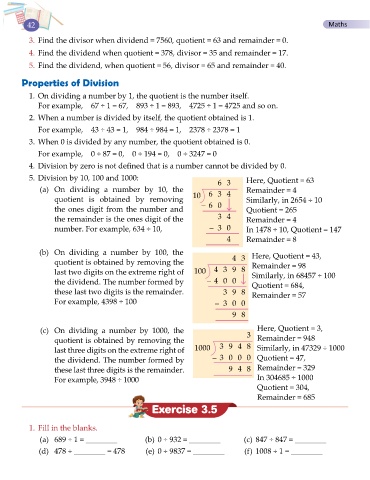
3. Find the divisor when dividend = 7560, quotient = 63 and remainder = 0.

4. Find the dividend when quotient = 378, divisor = 35 and remainder = 17.
5. Find the dividend, when quotient = 56, divisor = 65 and remainder = 40.

Properties of Division

1. On dividing a number by 1, the quotient is the number itself.
For example, 67 ÷ 1 = 67, 893 ÷ 1 = 893, 4725 ÷ 1 = 4725 and so on.
2. When a number is divided by itself, the quotient obtained is 1.

For example, 43 ÷ 43 = 1, 984 ÷ 984 = 1, 2378 ÷ 2378 = 1
3. When 0 is divided by any number, the quotient obtained is 0.
For example, 0 ÷ 87 = 0, 0 ÷ 194 = 0, 0 ÷ 3247 = 0

4. Division by zero is not defined that is a number cannot be divided by 0.

5. Division by 10, 100 and 1000: 6 3 Here, Quotient = 63
(a) On dividing a number by 10, the 6 3 4 Remainder = 4
quotient is obtained by removing 10 Similarly, in 2654 ÷ 10
the ones digit from the number and – 6 0 Quotient = 265
the remainder is the ones digit of the 3 4 Remainder = 4
number. For example, 634 ÷ 10, – 3 0 In 1478 ÷ 10, Quotient = 147
4 Remainder = 8

(b) On dividing a number by 100, the Here, Quotient = 43,
quotient is obtained by removing the 4 3 Remainder = 98
last two digits on the extreme right of 100 4 3 9 8 Similarly, in 68457 ÷ 100
the dividend. The number formed by – 4 0 0 Quotient = 684,
these last two digits is the remainder. 3 9 8 Remainder = 57
For example, 4398 ÷ 100 – 3 0 0

9 8

(c) On dividing a number by 1000, the 3 Here, Quotient = 3,
quotient is obtained by removing the Remainder = 948
last three digits on the extreme right of 1000 3 9 4 8 Similarly, in 47329 ÷ 1000
the dividend. The number formed by – 3 0 0 0 Quotient = 47,
these last three digits is the remainder. 9 4 8 Remainder = 329
For example, 3948 ÷ 1000 In 304685 ÷ 1000
Quotient = 304,
Remainder = 685
Exercise 3.5

1. Fill in the blanks.

(a) 689 ÷ 1 = ________ (b) 0 ÷ 932 = ________ (c) 847 ÷ 847 = ________
(d) 478 ÷ ________ = 478 (e) 0 ÷ 9837 = ________ (f) 1008 ÷ 1 = ________