Page 162 - Math Skill - 4

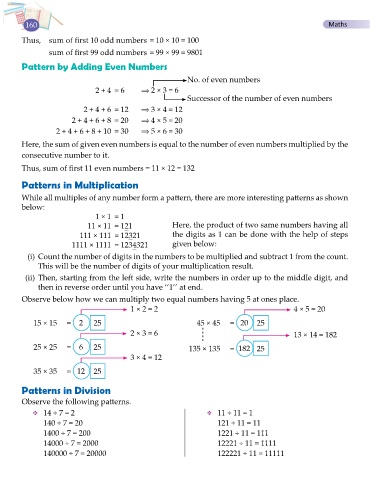
160 Maths

Thus, sum of first 10 odd numbers = 10 × 10 = 100

sum of first 99 odd numbers = 99 × 99 = 9801

Pattern by Adding Even Numbers
No. of even numbers

2 + 4 = 6 ⇒ 2 × 3 = 6
Successor of the number of even numbers
2 + 4 + 6 = 12 ⇒ 3 × 4 = 12

2 + 4 + 6 + 8 = 20 ⇒ 4 × 5 = 20
2 + 4 + 6 + 8 + 10 = 30 ⇒ 5 × 6 = 30

Here, the sum of given even numbers is equal to the number of even numbers multiplied by the
consecutive number to it.

Thus, sum of first 11 even numbers = 11 × 12 = 132

Patterns in Multiplication

While all multiples of any number form a pattern, there are more interesting patterns as shown
below:
1 × 1 = 1
11 × 11 = 121 Here, the product of two same numbers having all
111 × 111 = 12321 the digits as 1 can be done with the help of steps
1111 × 1111 = 1234321 given below:

(i) Count the number of digits in the numbers to be multiplied and subtract 1 from the count.
This will be the number of digits of your multiplication result.
(ii) Then, starting from the left side, write the numbers in order up to the middle digit, and
then in reverse order until you have ‘‘1’’ at end.
Observe below how we can multiply two equal numbers having 5 at ones place.
1 × 2 = 2 4 × 5 = 20

15 × 15 = 2 25 45 × 45 = 20 25

2 × 3 = 6 13 × 14 = 182
25 × 25 = 6 25 135 × 135 = 182 25
3 × 4 = 12

35 × 35 = 12 25

Patterns in Division

Observe the following patterns.
™ 14 ÷ 7 = 2 ™ 11 ÷ 11 = 1
140 ÷ 7 = 20 121 ÷ 11 = 11
1400 ÷ 7 = 200 1221 ÷ 11 = 111
14000 ÷ 7 = 2000 12221 ÷ 11 = 1111
140000 ÷ 7 = 20000 122221 ÷ 11 = 11111