Page 47 - Mathematics Class - IX

Similarly, superimpose ∆PQR on ∆STU completely only under the correspondence P ↔ S, Q ↔ T and
R ↔ U. See that ∆PQR covers ∆STU completely.

Hence, ∆PQR ≅ ∆STU if ∠Q = ∠T, QR = TU and ∠R = ∠U.
This is ASA criterion for congruency.

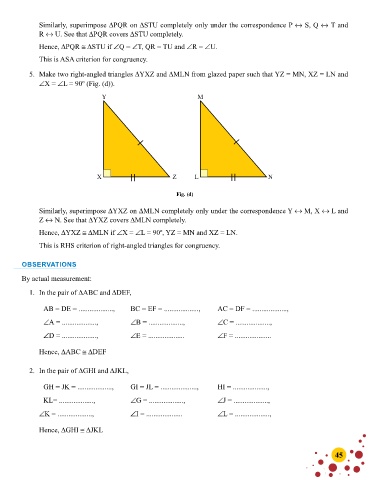
5. Make two right-angled triangles ∆YXZ and ∆MLN from glazed paper such that YZ = MN, XZ = LN and
∠X = ∠L = 90º (Fig. (d)).

Y M

X Z L N

Fig. (d)

Similarly, superimpose ∆YXZ on ∆MLN completely only under the correspondence Y ↔ M, X ↔ L and
Z ↔ N. See that ∆YXZ covers ∆MLN completely.

Hence, ∆YXZ ≅ ∆MLN if ∠X = ∠L = 90º, YZ = MN and XZ = LN.

This is RHS criterion of right-angled triangles for congruency.

OBSERVATIONS
By actual measurement:

1. In the pair of ∆ABC and ∆DEF,

AB = DE = ..................., BC = EF = ..................., AC = DF = ...................,
∠A = ..................., ∠B = ..................., ∠C = ...................,

∠D = ..................., ∠E = .................... ∠F = ....................

Hence, ∆ABC ≅ ∆DEF

2. In the pair of ∆GHI and ∆JKL,

GH = JK = ..................., GI = JL = ...................., HI = ...................,

KL= ..................., ∠G = ..................., ∠J = ...................,
∠K = ..................., ∠I = .................... ∠L = ...................,

Hence, ∆GHI ≅ ∆JKL

45