Page 55 - Mathematics Class - XII

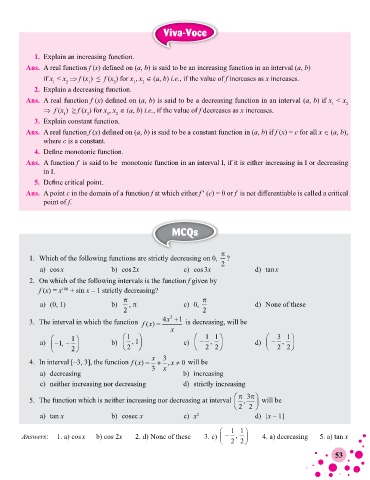
Viva-Voce

1. Explain an increasing function.
Ans. A real function f (x) defined on (a, b) is said to be an increasing function in an interval (a, b)
if x < x ⇒ f (x ) ≤ f (x ) for x , x ∈ (a, b) i.e., if the value of f increases as x increases.
1 2 1 2 1 2
2. Explain a decreasing function.

Ans. A real function f (x) defined on (a, b) is said to be a decreasing function in an interval (a, b) if x < x
2
1
⇒ f (x ) ≥ f (x ) for x , x ∈ (a, b) i.e., if the value of f decreases as x increases.
1 2 1 2
3. Explain constant function.
Ans. A real function f (x) defined on (a, b) is said to be a constant function in (a, b) if f (x) = c for all x ∈ (a, b),
where c is a constant.
4. Define monotonic function.
Ans. A function f is said to be monotonic function in an interval I, if it is either increasing in I or decreasing
in I.
5. Define critical point.

Ans. A point c in the domain of a function f at which either f ′ (c) = 0 or f is not differentiable is called a critical
point of f.

MCQs

p
1. Which of the following functions are strictly decreasing on 0, ?
a) cos x b) cos 2x c) cos 3x 2 d) tan x

2. On which of the following intervals is the function f given by
f (x) = x + sin x – 1 strictly decreasing?
100
p p
a) (0, 1) b) , p c) 0, d) None of these
2 2
2
3. The interval in which the function fx() 4 x 1 is decreasing, will be
x

3 1
1 1
a) 1, 1 b) 1 1 , c) , d) ,

2 2 2 2 2 2
x 3
4. In interval [–3, 3], the function fx() x , 0 will be
3 x
a) decreasing b) increasing
c) neither increasing nor decreasing d) strictly increasing
5. The function which is neither increasing nor decreasing at interval will be

,
22
a) tan x b) cosec x c) x d) | x – 1 |
2
1 1
Answers: 1. a) cos x b) cos 2x 2. d) None of these 3. c) , 4. a) decreasing 5. a) tan x

2 2
53